Remembering Socrates

When asked whether the square root of 2 can be written as an exact fraction, i.e., as the ratio of two integers, many people will say they don't know. This has always seemed interesting to me, because of course they do know - which is to say, they are in possession of all the information and understanding necessary to know the answer. If the square root of 2 equals M/N then 2 = (M/N)², and if they remember that numbers factor uniquely into primes, it's immediately obvious that 2N² = M² is impossible, because a square can't equal twice a square. (The exponent of the prime 2 in M² is even, whereas the exponent of 2 in 2N² is odd.)

Even without invoking unique factorization, Euclid described a simple proof "from scratch" that anyone can easily follow. If the square root of 2 equals M/N for some integers M,N, then 2 = (M/N)², and we can assume that M,N are not both even, because if they were we could divide them both by 2 while preserving the ratio. Thus, at most one of M,N is even. Writing the equation in the form 2N² = M², we see that M² is even, and we also know that the square of an odd number is odd, so M itself must be even, and therefore N must be odd. Now, since M is even, there is an integer m such that M = 2m, so we can substitute this M into the equation 2N² = M² to give 2N² = 4m², which implies N² = 2m². But this shows that N², and therefore N, must be even, contradicting the fact that there must be a solution with M,N not both even.

It's interesting that nearly this very same example was used by Socrates to illustrate the same point, i.e., how people know more than they think they do. In Plato's "Meno" he recounts a dialogue between Socrates and a fellow Athenian on the subject of whether virtue can be taught. A recurring theme in Socrates' thought was that all the knowledge we are capable of possessing is already within us, and the process of reasoning something out is really just an act of recollection, i.e., remembering things we already (in some sense) know.

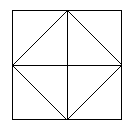
To illustrate this, Socrates questions an un-schooled servant boy about a simple geometrical proposition. Socrates draws a square, and asks the boy how he would go about constructing a square twice as large. Initially the boy says he doesn't know, but under further questioning he thinks the answer is to make the edges twice as long as the edges of the original square, making a figure like this:

But then Socrates asks him how much area this new square covers in relation to the original, and the boy correctly observes that it has four time the area. So Socrates re-iterates the question: how would you construct a square with just twice (not four times) the area of the original? Obviously we need a square with half the area of the one just constructed. Socrates asks the boy if we can cut each of the four squares in half by drawing a line connecting opposite corners, and the boy answers Yes. They draw these lines ("clever men call this the diagonal") and arrive at the figure below:

They agree that the four diagonals describe a square, and its area is twice the area of the original square.

Socrates:        What do you think, Meno?  Has he, in his answers, expressed any opinion that was not his own?

Meno:            No, they were all his own.

Socrates:        And yet, as we said a short time ago, he did not know?

Meno:            That is true.

Socrates:        So these opinions were in him, were they not?

Meno:            Yes.

Socrates:        So the man who does not know has within himself true opinions about the things that he does not know?

Meno:            So it appears.

Socrates:        These opinions have now just been stirred up like a dream, but if he were repeatedly asked these same questions in various ways, you know that his knowledge about these things would be as accurate as anyone's.

Meno:            It is likely.

Socrates:        And he will know it without having been taught, but only questioned, and find the knowledge within himself?

Meno:            Yes.

Socrates:        And is not finding knowledge within oneself recollection?

Socrates then goes on to speculate on when or how the boy had acquired his true opinions about geometry, and suggests that it must not have been during his present life (since Meno assures Socrates that the boy has had no instruction in geometry).

Of course, we might observe that what clever men call the line connecting opposite corners ("diagonal") was not one of the boy's own opinions. He was taught this by Socrates, so one could argue that the boy has in fact been taught something which he did not know, and which he (presumably) could never "recollect" simply by examining his opinions in isolation. This highlights two different kinds of knowledge, one that derives uniquely from first principles (the common notions about geometrical shapes) and the other that is accidental and arbitrary (terminology). More fundamentally, one could argue that people DO learn and acquire common notions about spatial relations and proportions during their formative years, as they organize their primitive sense perceptions. On the other hand, certain very basic aspects of our experience may be "hard-wired" into the biology of our brains and sense organs. This seems to be a mode of transmission for information that Socrates doesn't consider.

To his credit, Socrates concludes

I do not insist that my argument is right in all respects, but I would content... that we will be better men, braver and less idle, if we believe that one must search for the things one does not know...

Incidentally, the very next section of the "Meno" dialogue contains another geometrical example that is raised by Socrates to make a point about whether knowledge is teachable. Unfortunately the exact sense of his words is unclear, and the available translations are all slanted toward one particular interpretation or another. Thomas Heath says that this example is

much more difficult [than the previous example], and it has gathered round it a literature almost comparable in extent to the volumes that have been written to explain the Geometrical Number of the Republic. C. Blass, writing in 1861, knew thirty different interpretations; and since then many more have appeared. Of recent years, Benecke's interpretation seems to have enjoyed the most acceptance; nevertheless I think that it is not the right one...

Heath then goes on to give the interpretation that he thinks most closely fits the text (based on ideas of S. H. Butcher and E.F. August). However, it seems to me that Heath's proposed interpretation is not much more persuasive than any of the others for exactly what Socrates (or Plato) had in mind.

The translation of Plato's text available from most sources today is based on Heath's interpretation. Here is how Heath thinks the passage should be read:

If we are asked whether a specific area can be inscribed in the form of a triangle within a given circle, [we] might say... if that area is such that when one has applied it as a rectangle to the given straight line in the circle it is deficient by a figure similar to the very figure which is applied, then [we have our answer].

This is not abundantly clear. Heath gives a somewhat mundane reconstruction based on ordinary rectangles and triangles on the diameter of the circle, and his explanation is nominally plausible (under the interpretation he provides). However, Socrates' peculiar description has always reminded me of something else entirely.

Recall that Plato became a pupil and friend of Socrates in 407 BC, and Socrates himself lived from 469 to 399 BC. One of the most striking geometrical results of Greek mathematics was the quadrature of the lune, accomplished by Hipppocrates around 440 BC. This would have been one of the most talked-about results during the years when Socrates was beginning his teaching, because it was the first time anyone was able to construct, by classical methods, the area of a region with a curved boundary.

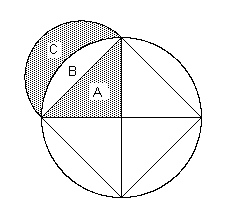
Moreover, it connects directly to the simple example of "doubling the square" that is discussed earlier in Meno, as can be seen from the drawing below:

The key to Hippocrates' argument is that the quadrant of the main circle (consisting of the regions A and B) obviously has 1/4 the area of the main circle. Also, the smaller circle has a diameter equal to 1/√2 of the larger circle, because it is what clever men call the diagonal of a square whose sides are half of the main circle's diameter. Consequently we know that the smaller circle has exactly half the area of the larger circle, which implies that the smaller half-circle (the regions B and C) has exactly the same area as the larger quarter-circle (the regions A and B). Hence we have A + B = B + C, and so A = C. In other words, the area of the "lune" (region C) equals the area of the inscribed triangle A.

In other words, the area of the lune is inscribed as a simple triangle in the circle if, when we construct a circle on the edge of that triangle, the region that is excluded from the main circle is equal to the area of the inscribed triangle. Also, the triangle is deficient (relative to the quadrant of the circle) by the very same shape by which the smaller semi-circle exceeds the required area. Recall Heath's translation of Plato's account of Socrates' dialogue

 ... a specific area can be inscribed in the form of a triangle within a given circle... if that area is such that when one has applied it ... to the given straight line in the circle it is deficient by a figure similar to the very figure which is applied, then [we have our answer].

Here I've omitted the phrase "as a rectangle". Without going back to the ancient Greek text, it's difficult to say how each term and phrase was intended, and words like "similar" vs "equivalent" are often up to the translator to decide based on his understanding of the context. Heath's translation was naturally slanted toward his own guess as to what mathematical construction Socrates was describing, whereas other scholars have read and translated the same text differently. It would be interesting to return to the original Greek text, with quadrature of the lune in mind, to see if a translation based on this interpretation is feasible.

Return to MathPages Main Menu