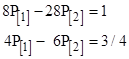On the Correlations of Entangled Particles

In this department of physics, in which we can make no progress without some hypothesis that looks somewhat startling at first sight, we must be careful not rashly to reject a new idea…

                                                                                                        Lorentz, 1909

Introduction

A simple example of Bell’s inequality can be based on spin-1/2 particles passing through Stern-Gerlach devices that can be oriented independently to measure the spins of the particles along any one of three angles, at 0, 120, and 240 degrees. When the measurement angles for a given pair of entangled particles are the same the results (spin up or down) are always opposite, whereas if the measurement angles are different the results agree 3/4 of the time. As Bohr said, if you aren’t shocked by this, you haven’t understood it.

Assuming that the particles are completely separate after they are emitted, and that the choices of measurements angles at each spacelike-separated detector are arbitrary and independent, the perfect disagreement at equal measurement angles seems to imply that each particle must be pre-programmed to give a specific result, up or down, for each of the three measurement angles. Hence each pair can be characterized by three “bits” of information, such as {udd} representing the prepared results of the particle sent to Detector 1, in which case the prepared results of the entangled particle sent to Detector 2 must be {duu}. Hence there are eight possible kinds of pairs, which we denote as{uuu},{uud},{udu},{udd},{duu},{dud},{ddu}, and {ddd}. The probabilities of agreement (both up or both down) according to quantum mechanics for the nine possible combinations of measurement angles are shown below.

As one would expect, the overall probability of agreement for randomly chosen measurement angles is exactly 1/2. However, if we hypothesize that each of the eight possible kinds of pairs have probability 1/8, the probability of agreement for unequal measurement angles (the off-diagonal cells in the table above) would be just 1/2, and the overall probability of agreement for random measurement angles would be just 1/3, so that hypothesis is ruled out. Even if we assume the probabilities of {uuu} and {ddd} are zero and the remaining six kinds of pairs each have probability 1/6, the probabilities of agreement for unequal measurement angles would be just 2/3 (instead of 3/4), and would give only 4/9 (instead of 1/2) for the overall probability of agreement for randomly chosen measurement angles.

In fact, no assignment of probabilities to the eight possible (and assumed mutually exclusive) kinds of pairs can yield the experimentally verified quantum mechanical results. To prove this, note that, for measurements made at 0° and 120°, agreement will be found precisely for pairs of the types {ud*} and {du*} where “*” signifies either ‘u’ or ‘d’. (Recall that the opposite particle for each type has the opposite prepared spin results.) Thus we require

Similarly the probabilities of agreement for measurements at 0° and 240°, and for measurements at 120° and 240° degrees, respectively imply that

Adding these three together gives

Thus the sum of just these six probabilities equals 9/8, which exceeds 1, so this is impossible. For this reason, it appears that the response of the particles to the possible choices of measurements at spacelike-separated locations exhibit a degree of correlation that is inexplicable by any account of this kind – with the stated assumptions. Many different attempts have been made, by both physicists and philosophers, to rationalize this “non-classical” correlation.

Local and Global Outcomes

There are two kinds of “results” involved in this situation, the local up/down results at each measurement location, and the global agree/disagree results for the entangled pairs. The local results for each measurement always have the unconditional probability 1/2 of being spin up, regardless of the measurement angle, and regardless of what has happened, is happening, or will happen at the other measurement location. The entanglement is manifest only in the global correlations between the measurement angles and outcomes (agreement or disagreement) at the two spacelike-separate detectors. Of course, the existence of correlation between the measurement results for entangled particles is not, in itself, surprising, since both particles emanate from the same emission event and hence classically can carry correlated (or anti-correlated) properties. Even the fact that the degree of correlation depends on the difference between the measurement angles is easily understandable in simple classical terms. For example, we saw above how the entangled pairs could be classically prepared to give the unconditional probability 1/2 for spin up at any given angle, and to yield perfect disagreement when measured at the same angles, and to give 2/3 agreement when measured at unequal angles. This gives probability of 4/9 for agreement if the measurement angles are chosen randomly. These are the maximum amounts of correlation (for unequal angles) that can be given by our simple classical model (given perfect anti-correlation at equal angles), and yet they fall short of the quantum mechanical predictions.

Uncertainty, Counterfactual Definiteness, and Probability

One basic premise of our analysis is that each particle of an entangled pair has precisely one of the eight preparations at emission, and is prepared to yield a definite spin for each of the three possible measurement angles. However, those are complementary observables, i.e., they do not commute, so the wave function of quantum mechanics doesn’t represent definite values for those observables beyond what is allowed by the uncertainty principle. Hence our premise amounts to the hypothesis that quantum mechanics provides an incomplete description of the particles (a hypothesis that is often associated with the idea of “hidden variables”). Indeed this was one of the points made in the 1935 paper of Einstein, Podolsky, and Rosen.

The classical analysis (based on the hypothesis of “hidden variables”) assumed that the eight possible types of pairs are mutually exclusive, but one could question this assumption. It’s fairly unobjectionable that the probability of {ud* or du*} is equal to the probability of ud* plus the probability of du*, because these are distinct measured outcomes, but it’s slightly less clear that the probability of ud* is equal to the sum of the probabilities of udu and udd. This latter assumption is based on the premise that the probability of {udu and udd} is zero, but the spin at 240° is not measured for either particle in this case, so it’s tempting to wonder if there might be a sense in which {udu and udd} has non-zero probability. This might be ruled out on the grounds that it would require the particles to “know” (when they are emitted) which measurement angles will subsequently be chosen.

Still, it’s worth noting that the empirical meaning of probability (at least in the frequentist interpretation) is based on the idea that we conduct a sequence of trials with certain conditions and determine the frequency of occurrence of the various outcomes. In this case the very conditions we are evaluating stipulate that we never distinguish between udu and udd, because we never measure the spin at 240° on either particle. (This is somewhat reminiscent of the need, pointed out by Bose, to use statistics that account for the identicality of some particles for quantum phenomena.) We assume that each pair of the type {ud*} must be either {udu} or {udd}, but not both, similar to how we may be tempted to assume that each particle in a two-slit experiment must pass through one slit or the other. In the case of the two-slit experiment the interference effects undermine our confidence in this assumption. Likewise we might regard the quantum correlations as “interference effects”.

To give a simplistic example, suppose that each of the eight possible types of pairs has absolute probability P_[1] and furthermore that the probability that a given pair of particles is of any two types is P_[2]. We may also assume that the probabilities of any three or more types is zero. On this basis, requiring that the union of all eight types is 1 and the probability of the union of four types is 3/4, we have

From this we get P_[1] = 15/64 and P_[2] = 1/32. This would apply if every intersection of two types had the same probability, but in a more refined example we might restrict this to pairs of types whose differences are never manifested by any interaction, so they can be regarded as non-distinct, and hence “both can occur”. However, this would seem to necessitate that the selection of the type(s) is informed by the eventual interactions.

Local and Global Information

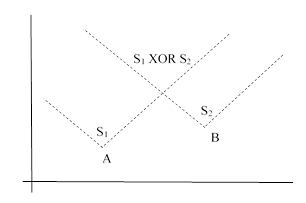
We need to distinguish between non-local facts and non-local evolution. Ironically, the principle of locality itself, combined with either randomness or free will, entails the existence of non-local facts, meaning that spacelike-separated observables commute. There are so many related assumptions that it’s difficult to unravel them and make statements about any specific assumption in isolation, but if we imagine (for example) some genuine randomness (events not implicit in antecedent events), then new information can be created, and this can be done at spacelike-separated events. If we create a string S₁ of a million random digits at location A and another string S₂ of a million random digits at location B, then S₁ is local information that propagates into the future lightcone of A, and S₂ is local information that propagates into the future lightcone of B, but there is also (arguably) the global information consisting of the string [S₁ XOR S₂]. When, or at what event, does this information “come into existence”? Surely it cannot be said to “exist” until after the second of the two strings has been formed, but both strings have definitely been formed only for events in the future light cones of both A and B, which is to say, events such that A and B are both in the past lightcones, as depicted below.

We typically don’t regard the XOR of the two strings as non-local information existing outside the future light cones of A and B because it has no physically detectable existence outside of that intersection. However, suppose the XOR of the two strings is found to be “11111…”, i.e., the two strings when compared are found to be perfect complements of each other. Admittedly there is a non-zero probability of this occurring by chance, and even for it to continue occurring on arbitrarily many repeated trials, but we discount this explanation. (We might call this a perpetual miracle - Leibniz’s phrase to describe Newton’s theory of gravity.) Would we conclude that the generation of the strings was not random, or that it was not independent?

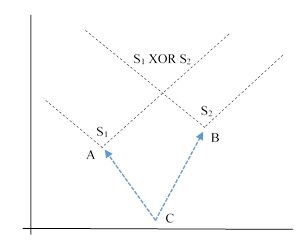
One might naively imagine that the “first” string was random, and then by some superluminal conveyance of information the second string is produced by a totally deterministic process, making it select the complement of the bit in the first string. However, from a Lorentz-invariant perspective we can’t say whether A or B occurs first, because each of them is “first” in some system of inertial coordinates. The naïve hypothesis asks us to believe that, for two seemingly identical random number generators, one is actually perfectly random whereas the other is perfectly deterministic, producing the complement bits by some incorporeal influence. Clearly the two are symmetrical, so this naïve attempt to view the situation in the context of a preferred frame would be misguided (introducing asymmetries not inherent in the phenomena, to use Einstein’s phrase). Of course, we would discount this entirely if we knew that pairs of particles are emitted from a common past event C (with a single random number generator) and arriving at events A and B as shown below.

In this situation we would naturally deduce that the complementary random bits appearing at A and B are both generated at the common event C in the past, and these bits of information are conveyed to B and C by the particles.

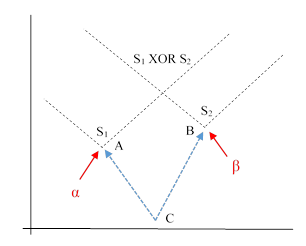
With that hypothetical background, consider an actual EPR experiment involving quantum entanglement. The additional feature is that some “external information, consisting of the choice of measurement angle, arrives at each detector A and B, as shown below.

If α and β have the same values (meaning the same measurement axis is selected for both particles), the situation is identical to what was described previously. The strings S₁ and S₂ are exact complements, and we would likewise attribute this to the common preparation of the particles emanating from C. However, if for a given pair of particles the measurement angles selected by α and β are different, the bits agree 3/4 of the time. The difficulty in reconciling this with the perfect anti-correlation at equal angles by means of classically prepared mutually exclusive pair types was described above, but the distinction to be made here concerns the time and place at which we can say the non-classical correlations “come into existence”. In the example with no correlation at all, it seemed acceptable to regard the XOR of the two strings as existing only in the intersection of the forward lightcone of the measurements, whereas when we stipulated perfect anti-correlation we could rationalize it classically only by supposing that the anti-correlation came into existence at the common emission event C. But the 3/4 agreement at unequal angles seems to invalidate the classical account of the bit correlations being established at C. This leads to the impression that the XOR of the two strings must already exist in a non-local way outside the intersection of the forward light cones of the measurements, even though for this region there are inertial frames of reference in terms of which one of the measurements has not even occurred yet.

Determinism

Bell contributed to the confusion in this subject by inventing the word “superdeterminism” to describe the possibility that the choice of measurement angles at the detectors may not be independent. He allowed that if this were the case, then a local realistic account of the correlations could not be ruled out. This is true, but the word “superdeterminism” is misleading, because there is really only one kind of determinism. The concept to which Bell alluded is that there could be non-local correlations between the measurement angles (a and b in our terminology), and these might arise deterministically through a purely local law of evolution. One possibility is that the correlations in measurement angles might arise from the common causal past of those measurements (such as during the brief time that the emitter is preparing to emit the particles), but it’s possible to imagine that the measurement angles are selected by signals come from arbitrarily far apart (in an infinite flat universe) so that they don’t share a common past. This, however, entails cosmological challenges to explain the uniformity of the universe if parts of the universe really have never been in contact. Another possibility is that the initial conditions of the universe (Cauchy slice zero) already exhibited certain global (non-local) symmetries, and these symmetries are thereafter propagated by a purely local law of evolution.

Everyone is familiar with at least a mild example of this.  Recall that in general relativity we don’t have complete freedom to define the field on an initial Cauchy surface (as we do with electromagnetism, for example).  This is because the initial field must everywhere satisfy a non-trivial constraint, namely, the Bianchi identities.  Given an initial Cauchy surface that satisfied these conditions, the field equations of general relativity ensure that the conditions continue to be satisfied on all subsequent Cauchy surfaces.  No one refers to general relativity as a “superdeterministic” theory just because its initial conditions are constrained. Of course, the Bianchi identities are a local constraint, so it isn’t surprising that they are preserved by the local field equations. The question is whether non-local symmetries in the initial conditions could be preserved by a purely local law of evolution.  This seems logically possible (to me).  We can see this in cellular automata, for example.  It isn’t an inherently silly idea, and it certainly doesn’t render science itself impossible.  After all, we only need the theory to preserve the symmetries that actually exist.

There have been several proposed “explanations” of the non-classical correlations implied by quantum entanglement, including Copenhagen, many worlds, undetectable non-local many-fields (also known as Bohmian mechanics), complete determinism with local evolution preserving initial symmetries, and so on.  Each interpretation has been branded as unscientific (and worse) by everyone except the proponents of that particular interpretation.

Minkowskian Locality

One major source of confusion in this subject is the widely varying conceptions and usages of the word “local”.  Bell was responsible for popularizing the word non-locality to describe quantum entanglement, but (again) his choice of terminology was bad. It was, however, understandable, because Bell actually did believe that Lorentz invariance was violated, and that “behind the scenes” something really was moving faster than light.

Another related source of confusion is that Bell and others generally spoke (and thought) in terms of non-relativistic quantum mechanics, which of course is non-relativistic, because the non-relativistic Schrodinger equation is not Lorentz invariance, not involving the constant c, and a massive particle (for example) can be accelerated to arbitrary speeds, so obviously superluminal communication is permitted in this non-relativistic context.  Hence, discussing the question of whether nature is “local” in the context of non-relativistic quantum mechanics makes no sense, since that context is inherently non-local, and therefore wrong. We know that relativistic quantum mechanics is required more correct, and of course it is relativistic. These statements sound tautological, but a surprising number of people seem to overlook this. Part of the reason may be that even non-relativistic quantum mechanics entails quantum entanglement, and this entanglement in itself does not permit superluminal signaling, so people imagine that this implies we can safely consider locality in this framework, but that reasoning is obviously fallacious.

We’ve already commented on the important distinction between local facts versus local laws of evolution.  When asserting that nature is or is not “local” we need to specify which of these we have in mind. But more fundamentally, some people define locality as the condition that spacelike separated observables commute, or the proposition that no energy or information can propagate faster than light. But even the meaning of this is disputed (in some circles), because some people argue that the non-classical correlations exhibited by entangled systems imply superluminal and directional conveyance of information, even though the correlations do not represent any communication or signaling, and even though the fundamental asymmetry that such directional conveyance would imply for manifestly symmetrical phenomena makes this proposal incoherent. If two simultaneous measurements are simultaneously “informed”, then this is not directional conveyance, it is more properly to be regarded as non-separability.

Another important source of confusion is failure to account for the actual pseudo-metrical structure of spacetime when defining locality. In a topological manifold with a metric the concept of locality relates to neighboring points, based on the metrical closeness. We are most accustomed to metrical manifolds with a positive-definite metric, and such metrics induce the local Euclidean topology, with transitive locality, meaning that if A is close to B, and B is close to C, then A is close to C. But we know that the spacetime we inhabit does not have a positive-definite metric, it has the Minkowski metric, and we should really conceive of locality in terms of the sense of “closeness” induced by this (pseudo)metric.  Of course, this implies that locality is not transitive, because we can have A arbitrarily close to B, and B arbitrarily close to C, while A and C are arbitrarily far apart. It’s also noteworthy that the metric with its induced locality is temporally symmetrical.

In relativistic quantum mechanics, and quantum field theory, the relevant metric is the Minkowski metric.  This is the absolute interval that appears in the laws of physics. In particular we note that photons propagate essentially along null intervals, and the quantum wave function does not advance along a null interval. Even for EPR experiments there are always events that are null separated from the emission event and both distant reception events, even though those reception events are spacelike-separated from each other. Hence it is conceivable that the emission of the entangled particles is conditioned by the reception events, and this conditioning fully respects the Minkowskian locality of spacetime, by acting along null intervals.

Return to MathPages Main Menu