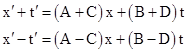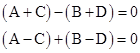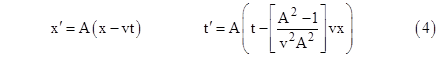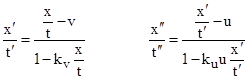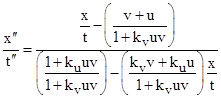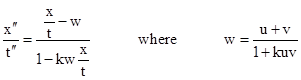Simple Derivation of Lorentz Transformation

Since inertia-based coordinate systems, by definition, map continuous straight loci to continuous straight loci (principle of inertia), the transformation for any given relative speed v is linear, meaning that it is of the form

for constants A,B,C,D for any given v. If we stipulate that the light-like loci x = ±t map to the loci x′ = ±t′ respectively, then from the relations

it follows that for any non-zero x such that x = −t the first expression vanishes, and for any non-zero x such that x = t the second expression vanishes, so we have

By adding and subtracting these, we get A=D and B=C. Also, at x′ = 0 we have 0 = Ax + Bt and hence B = −Av where v is the velocity of the spatial origin of the primed coordinates with respect to the unprimed coordinates. Therefore the transformation is of the form

Using these expressions for x′ and t′, we have A(vx′ + t′) = A²(1−v²)t and A(x′+vt′) = A²(1−v²)x, and therefore, since the principle of relativity requires that the inverse transformation must be identical to the above transformation, except with v replaced by –v, we must have A²(1−v²) = 1, and so

which completes the derivation.

The above derivation, like those in many traditional sources, follows Einstein’s 1905 decision to base the derivation on the invariant light-speed principle, expressed by the fact that the loci x = ±t map to x′ = ±t′. That’s an extremely restrictive premise and it makes the derivation quite simple. However, we can also proceed without that premise, noting that the algebraic inverse of (1) is

As before, we have B = −Av and B  = −Dv, from which we get A=D. Thus the inverse transformation can be written as

Again by the relativity principle we require that the inverse transformation be identical to the forward transformation except with –v in place of v, so the expression for x implies that the denominator must be 1, and hence we have C = (1−A²)/vA. Substituting the expressions for B, C, and D in terms of A back into (1), the transformation has the form

where A (remember) is a function of v. For any given v let k_v denote the quantity in the square brackets, so this can be written in the form

Every two standard inertial coordinate systems must be related in this way, so if we consider a third system x″,t″ with velocity u relative to x′,t′, we have

Substituting the expression for x′/t′ into the expression for x″/t″ and simplifying, we get

The first quantity in the denominator must equal 1, so we have k_u = k_v, meaning that the constant is the same for all values of the velocity, so we can just call it k. Also, the second quantity in the numerator must be the relative velocity w between x,t and x″,t″, and hence we have

consistent with the principle of relativity. Thus we have found, purely from the principle of relativity (along with isotropy and homogeneity to yield linearity) that any two systems of coordinates in terms of which the equations of Newtonian mechanics hold good in the low speed limit (i.e., the equations of physics take the same homogeneous and isotropic form) must be related by a transformation of the form

where k is some universal constant with units of inverse squared velocity. Notice that we have not made any use of an assumption about the speed of light. This form of the transformation is implied purely by the existence of standard inertial coordinate systems with the defining property. It only remains to determine the value of k. By a suitable choice of units for space and time we could normalize the value of k to be either −1, 0, or +1. If k = 0 then these equations yield the Galilean transformations, whereas if k = −1 they yield the Euclidean rotations in the x,t plane. If k=1 they yield the Lorentz transformation. It can be shown that the energy E has inertia corresponding to kE, so from the experimental fact that every quantity of bound energy E has rest mass E/c² it follows that k=1/c². This is such a small quantity that it is understandable why it was mistaken for zero prior to experiments with high speed particles in the early 1900s. According to Newtonian mechanics an object subjected to a constant force would undergo constant acceleration indefinitely, but we actually find that objects accelerate more slowly at high speeds, and they asymptotically approach the speed c. This fundamentally demonstrates the Lorentz invariance of the laws of physics. In fact, any experimental result that implies k=1/c² suffices.

We can also carry the a prior approach still further, and adduce rational arguments for why k=1 is a logically necessary fact, as we’ve discussed elsewhere, but the above discussion represents the two most common approaches to economical derivations of Lorentz invariance.

Return to MathPages Main Menu