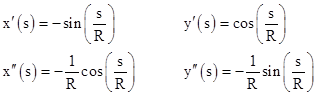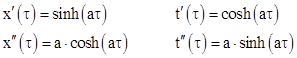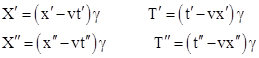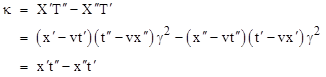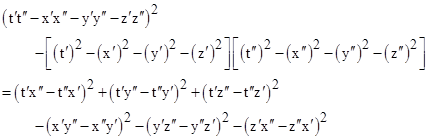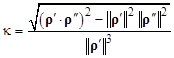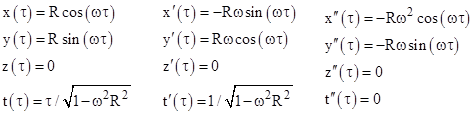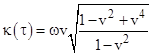Curvature of Paths in Spacetime

Given a system of Cartesian coordinates x,t on the Euclidean plane, let x(s) and y(s) be parametric equations of a curve, where s is a metrical path length parameter along the curve. As derived in another note, the extrinsic signed curvature is given by

where primes signify derivatives with respect to s. A simple example is a circular path defined by

The arguments of the sine and cosine are s/R because they represent the central angle θ that ranges from 0 to 2π as the circumferential path length s ranges from 0 to 2πR. For this path we have

and hence the signed curvature is

So, as expected, the extrinsic curvature at every location along this circular path of radius R has the constant value 1/R. Naturally the extrinsic curvature of a trajectory at a given point is invariant under any translations or re-orientations of the coordinates. We can also imagine placing a circle tangent to a curve (in the osculating plane) at a given point, and varying the radius of the circle until it matches the curve to second order at that point, and since this matching is maintained by a smooth transformation, and the curvature of the circle is invariant, it follows that the curvature of any curve is invariant under translation and re-orientation of the coordinate system.

In terms of standard inertial coordinates x,t in space and time we can consider a timelike path of constant proper acceleration, which represents hyperbolic motion with the parametric equation (in geometrical units, so c=1)

where τ is the proper time along the trajectory, i.e., the pseudo-metrical path length parameter. For this path we have

Based on this we find that the same formula for the signed curvature gives

This shows that the extrinsic curvature at every location along this hyperbolic path of constant proper acceleration “a” through spacetime has the constant value −a. This trajectory has the congruent shape when subjected to any Lorentz transformation, which simply causes a different event on the trajectory to be the event at which the curve is stationary. Naively one might be  surprised that the curvature along this hyperbola is constant, since when plotted in terms of x,t as if the metric was Euclidean it appears that the curvature is most extreme at the stationary point, and becomes asymptotically straight on the limbs. However, the proper time parameter τ is not equal to the Euclidean path length as drawn in this form. In the regions where the trajectory seems to become asymptotically straight, the rate of proper time becomes correspondingly reduced. This is consistent with the fact that every event on the trajectory is equivalent, and can be seen as the knee of a congruent hyperbola (just as every point on a Euclidean circle is equivalent).

As with the circle in Euclidean space, we can imagine placing a hyperbola of constant proper acceleration tangent to any other timelike curve (in the osculating plane) at a given event, and varying the acceleration until it matches the curve to second order at that point, and since this matching is maintained by a smooth transformation, and the curvature of the hyperbolic path is invariant, it follows that the curvature of any timelike trajectory in spacetime is invariant under Lorentz transformations and translations of the coordinate system.

To show this explicitly, consider an arbitrary timelike trajectory with the parametric expressions for x(τ) and t(τ) in terms of standard inertial coordinates x,t, and apply a Lorentz transformation to give the parametric expressions X(τ) and T(τ) in terms of a relatively moving system of standard inertial coordinates X,T. Naturally the proper time τ along the path is invariant. Now, for any given v, the transformed expressions are

where γ = 1/(1 − v²)^1/2, and the derivatives with respect to τ are

In terms of the X,T coordinates the extrinsic curvature is

which proves that the curvature is invariant.

It’s interesting that, making use of the Fibonacci identity, the basic expression for the curvature in 1+1 dimensions can be written as

Letting ρ denote the vector [t,x], we can also express this in terms of the Minkowskian inner product and squared norms as

Recall that we are using the path parameter τ, so no normalizing is needed. The same expression applies in 3+1 dimensional space-time with the displacement 4-vector ρ = [t,x,y,z], as shown by the identity

To allow for an arbitrary scalar parameter, instead of the path length parameter, the general expression for the extrinsic curvature of a trajectory in spacetime includes the normalizing factor in the denominator, so we have

The inner product of any 4-vectors is invariant under Lorentz transformation, so this makes explicit the fact that the extrinsic curvature of any trajectory embedded in 3+1 dimensional space-time is invariant under Lorentz transformations.

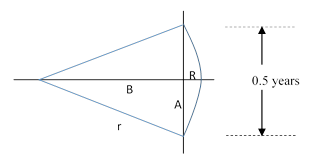
As an example, consider the helical path of the earth in its orbit around the Sun. Intuitively we would expect the radius of curvature of this trajectory to be between about one and two thousand light years. To see why, consider that in the approximate osculating plane of the earth’s trajectory in spacetime over a six month time interval the earth moves “sideways” by one orbital radius and then back, so the trajectory is approximately as shown below.

where R is the orbital radius of the earth and r is the radius of curvature of the earth’s helical trajectory in spacetime. Making use of the relations r − B = R and B = √(r² – A²), we have

Solving this for r gives

Taking all the quantities in units of light-years, we have A = 1/4 and R equals 8.3 minutes (time for light to travel from sun to earth) divided by the number of minutes in a year, or equivalently, 93 million miles divided by the number of miles in a light year. Thus R = 1.58E-05, so the radius of curvature would be expected to be or the order of 1978 light-years. However, in this rough assessment we neglected the curvature due to the varying osculating plane itself, so the actual curvature should be somewhat greater, and hence the radius of curvature somewhat smaller. Using the formula for curvature discussed previously, we can compute the exact value (1591 light years) as follows.

For simplicity, we regard the earth’s trajectory as a perfectly circular orbit with radius R and angular velocity ω = dθ/dτ, where τ is the proper time of the earth. Using units such that c = 1, we have the parametric expressions for the coordinates and their derivatives in terms of the standard inertial coordinate system in which the sun is at rest:

Inserting the first and second derivatives into the expression for the curvature, and putting v = ωR, the curvature of this helical trajectory has the constant value

Thus for values of v much less than 1 the curvature is essentially just ωv. The orbital speed of the earth in terms of these coordinates is about v = 10⁻⁴, so the curvature of the earth’s trajectory is about (2π)10⁻⁴ lightyear⁻¹, meaning that the “radius of curvature” is about 1591 light years. In terms of any other standard system of inertial coordinates, related to this system by a Lorentz transformation, the curvature of the trajectory is the same, as shown above.

Return to MathPages Main Menu