Planetary Alignment

If several planets are orbiting the sun at known speeds and current positions, how many years will it be before those planets are perfectly aligned? This is a frequently asked question, but it's a bit tricky, because it involves the issue gravitational harmonics, which tend to force the periods of the planetary orbits to be rational multiples of each other. However, setting this aside, and assuming arbitrary real constants for the periods of the planets, the answer is that - except in very special cases - the planets will never be perfectly aligned (assuming we have more than two planets).

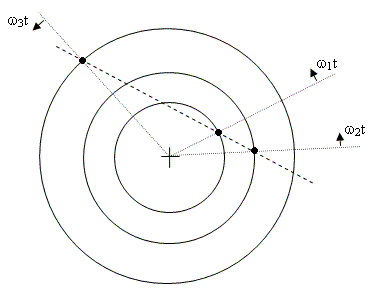
Consider the hands of a clock-like mechanism.  Suppose the hands are initially aligned at "12 o'clock", and the angular velocities of the first two hands are w₁ and w₂ radians/sec, respectively.  How often will those two hands be perfectly aligned?  This is equivalent to asking how often two planets will lie along a straight line through the sun (i.e., the center of the clock). The positions of the two hands at any time t are w₁ t and w₂ t, and they are aligned if and only if (w₁-w₂) t  is an integer multiple of 2p.  Therefore, the two hands are aligned at the time values given by

However, if we include a third hand on the clock, with angular velocity w₃, and assume it was also aligned initially at t = 0, then it will be aligned with the first hand at the times

All three hands will be aligned simultaneously only when both of these equations are satisfied, which implies  k (w₁-w₃)  =  j (w₁-w₂)  and so

Since j and k are integers, it follows that (w₁-w₃)/(w₁-w₂)  must be a rational number. But suppose

In this case the ratio (w₁-w₃)/(w₁-w₂) equals the square root of 2, which is irrational. Thus the three hands will never come into perfect alignment, except in the special case when all three speeds are rational multiples of each other (as in the case of a real clock mechanism). This corresponds to the fact that three planets with arbitrary real angular speeds will never again (after initially being aligned) all lie along a single line through the Sun.

However, it isn’t necessary to restrict our attention to alignments that include the Sun. We can typically find an infinite sequence of alignments between three planets, i.e., configurations in which the three planets lie along a single line, although this line does not pass through the Sun. This type of alignment is illustrated below.

Arbitrarily assigning the positions to be along the positive x axis at time t = 0, the positions of the three planets have the coordinates

A necessary and sufficient condition for the three planets to lie on a single line is that the slope of the line through planets 1 and 2 must equal the slope of the line through planets 2 and 3.  Thus the three planets are aligned if and only if

Clearing fractions and re-arranging terms, this is equivalent to the condition

This is just the signed area of the triangle formed by the planets. The vanishing of this area is equivalent to the three points being co-linear. Substituting from the previous expressions for the coordinates and simplifying the resulting equation, we find that the planets are aligned at every times t satisfying the condition

For perfectly circular orbits, Kepler’s third law is GM = w²r³ , so with appropriate choice of units for t and r we can express this entirely in terms of the three radii and the time as follows

If the radii are expressed in astronomical units (i.e., if r_e = 1 is the Earth’s orbital radius), then the units of time are

Of course, this alignment equation doesn’t account for orbital eccentricities, or for deviations of the orbits from the ecliptic, but it does give a general idea of the patterns of alignment that would be expected for planets with roughly circular orbits at those three radii.  To illustrate, let f(t) denote the left hand side of the alignment equation, with the radii r₁ = 0.387, r₂ = 0.723, and r₃ = 1, corresponding to the orbits of Mercury, Venus, and Earth. The function f(t) versus t is as shown below for a typical five-year period.

This shows that there are usually four discrete alignments of these three planets every 1.566 years. However, the function is not exactly periodic, as can be seen from the slight variations in the shape of the function from one “cycle” to the next. By examining a longer time scale, we can see that these variations themselves are cyclical, with a period of roughly 49.2 years. Once per period, the shoulder inflection point of f(t) passes through zero, so once every 49.2 years there is a period of about one month during which these three planets (if they were in idealized circular orbits), would be more or less continuously aligned. One such prolonged syzygy is shown in the plot below.

Midway between each of these prolonged syzygies is a switchover during which one zero-crossing pair disappears and another appears, as shown below.

Similarly we can evaluate the syzygy functions for other combinations of three planets. For example, the function for Venus, Earth, and Mars over a typical ten-year span of time is shown below.

This shows that the lowest distinct quasi-period for these three planets is over six years.

Exact linear alignments of four or more planets do not generally occur, but we can produce a function whose magnitude is a measure of the non-linearity of four planets by adding the squares of two three-planet syzygy functions.  For example, if f₁₂₃(t) is the function for Mercury, Venus, and Earth, and f234(t) is the function for Venus, Earth, and Mars, then the function

equals zero if and only all four planets are co-linear. Beginning with all four planets on the positive x axis at t = 0, the value of F(t) over the first ten years is shown below.

The first close approach to co-linearity occurs at 0.92 years. The arrangements of the four planets in relation to the Sun at this time is shown in the figure below.

This configuration has the feature that the other three planets are all in the same direction from the Earth, so they would all be in visible conjunction. An expanded view of F(t) near zero shows that the next close approach to perfect linearity occurs at 3.15 years, when the configuration is as shown below.

In this case the other three planets are all on one side of the Earth, but they are also aligned with the Sun, and Mars is on the opposite side of the Sun, so this impressive configuration would not be very visible from the Earth. The next extremely near approach to perfect co-linearity of these four planets occurs at about 6.385 years, when the planets are arranged as shown below.

In this case Mercury and Venus are on one side of the Earth, and Mars is on the other, so their co-linearity would not appear as a triple “conjunction”.

Return to MathPages Main Menu