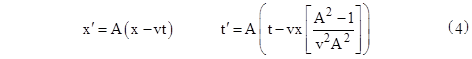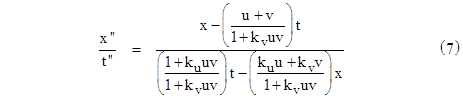Staircase Wit

 

Henceforth space by itself, and time by itself, are doomed to fade away into mere shadows, and only a kind of union of the two will preserve an independent reality.

                                                              H. Minkowski, 1908

 

In retrospect, it's easy to see that the Galilean notion of space and time was not free of conceptual difficulties. In 1908 Minkowski delivered a famous lecture in which he argued that the relativistic phenomena described by Lorentz and clarified by Einstein might have been inferred from first principles long before, if only more careful thought had been given to the foundations of classical geometry and mechanics. He pointed out that special relativity arises naturally from the reconciliation of two physical symmetries that we individually take for granted. One is spatial isotropy, which asserts the equivalence of all physical phenomena under linear transformations such as xʹ = ax – by, yʹ = bx + ay, zʹ = z, tʹ = t, where a2 + b2 = 1. It’s easy to verify that transformations of this type leave all quantities of the form x2 + y2 + z2 invariant. The other is Galilean relativity, which asserts the equivalence of all physical phenomena under transformations such as xʹ = x – vt, yʹ = y, zʹ = z, tʹ = t, where v is a constant. However, these transformations obviously do not leave the quantity x2 + y2 + z2 invariant, because they involve the time coordinate as well as the space coordinates. In addition, we notice that the rotational transformations maintain the orthogonality of the coordinate axes, whereas the lack of an invariant measure for the Galilean transformations prevents us from even assigning a definite meaning to “orthogonality” between the time and space coordinates. Since the velocity transformations leave the laws of physics unchanged, Minkowski reasoned, they ought to correspond to some invariant physical quantity, and their determinants ought to be unity. Clearly the invariant must involve the time coordinate, and hence the units of space and time must be in some fixed non-singular relation to each other, with a conversion factor that we can normalize to unity. Also, since we cannot go backwards in time, the space axis must not be rotated in the same direction as the time axis by a velocity transformation, so the velocity transformations ought to be of the form xʹ = ax – bt, yʹ=y, zʹ=z, tʹ = bx – at, where a2 – b2 = 1. Combining this with the requirements b/a = v, we arrive at the transformation

 

 

which leaves invariant the quantity x2 + y2 + z2 – t2. The rotational transformations also leave this same quantity invariant, so this appears to be the most natural (and almost the only) way of reconciling the observed symmetries of physical phenomena. Hence from simple requirements of rational consistency we could have arrived at the Lorentz transformation. As Minkowski said

 

Such a premonition would have been an extraordinary triumph for pure mathematics. Well, mathematics, though it now can display only staircase wit, has the satisfaction of being wise after the event... to grasp the far-reaching consequences of such a metamorphosis of our concept of nature.

 

Needless to say, the above discussion is just a rough sketch, intended to show only the outline of an argument. It seems likely that Minkowski was influenced by Klein’s Erlanger program, which sought to interpret various kinds of geometry in terms of the invariants under a specific group of transformations. It is certainly true that we are led toward the Lorentz transformations as soon as we consider the group of velocity transformations and attempt to identify a physically meaningful invariant corresponding to these transformations. However, the preceding discussion glossed over several important considerations, and contains several unstated assumptions. In the following, we will examine Minkowski’s argument in more detail, paying special attention to the physical significance of each assertion along the way, and elaborating more fully the rational basis for concluding that there must be a definite relationship between the measures of space and time.

 

For any system of mutually orthogonal spatial coordinates x,y,z, (assumed linear and homogeneous) let the positions of the two ends of a given spatially extended physical entity be denoted by x1,y1,z1 and x2,y2,z2, and let S2 denote the sum of the squares of the component differences. In other words

 

 

Experience teaches us that, for a large class of physical entities (“solids”), we can shift and/or re-orient the entity (relative to the system of coordinates), changing the individual components, but the sum of the squares of the component differences remains unchanged. The invariance of this quantity under re-orientations is called spatial isotropy. It’s worth emphasizing that the invariance of S2 under these operations applies only if the x, y, and z coordinates are mutually orthogonal.

 

The spatial isotropy of physical entities implies a non-trivial unification of orthogonal measures. Strictly speaking, each of the three terms on the right side of (1) should be multiplied by a coefficient whose units are the squared units of s divided by the squared units of x, y, or z respectively. In writing the equation without coefficients, we have tacitly chosen units of measure for x, y, and z such that the respective coefficients are 1.

 

In addition, we tacitly assumed the spatial coordinates of the two ends of the physical entity had constant values (for a given position and orientation), but of course this assumption is valid only if the entities are stationary. If an object is in motion (relative to the system of coordinates), then the coordinates of its endpoints are variable functions of time, so instead of the constant x1 we have a function x1(t), and likewise for the other coordinates. It’s natural to ask whether the symmetry of equation (1) is still applicable to objects in motion. Clearly if we allow the individual coordinate functions to be evaluated at unequal times then the symmetry does not apply. However, if all the coordinate functions are evaluated for the same time, experience teaches us that equation (1) does apply to objects in motion. This is the second of our two commonplace symmetries, the apparent fact that the sum of the squares of the orthogonal components of the spatial interval between the two ends of a solid entity is invariant for all states of uniform motion, with the understanding that the coordinates are all evaluated at the same time.

 

To express this symmetry more precisely, let x1,y1,z1 denote the spatial coordinates of one end of a solid physical entity at time t1, and let x2,y2,z2 denote the spatial coordinates of the other end at time t2. Then the quantity expressed by equation (1) is invariant for any position, orientation, and state of uniform motion provided t1 = t2. However, just as the spatial part of the symmetry is not valid for arbitrary spatial coordinate systems, the temporal part is not valid for arbitrary time coordinates. Recall that the spatial isotropy of the quantity expressed by equation (1) is valid only if the space coordinates x,y,z are mutually orthogonal. Likewise, the combined symmetry covering states of uniform motion is valid only if the time component t is mutually orthogonal to each of the space coordinates.

 

The question then arises as to how we determine whether coordinate axes are mutually orthogonal. We didn’t pause to consider this question when we were dealing only with the three spatial coordinates, but even for the three space axes the question is not as trivial as it might seem. The answer relies on the concept of “distance” defined by the quantity S in equation (1). According to Euclid, two lines intersecting at the point P are perpendicular if and only if each point of one line is equidistant from the two points on the other line that are equidistant from P. Unfortunately, this reasoning involves a circular argument, because in order to determine whether two lines are orthogonal, we must evaluate distances between points on those lines using an equation that is valid only if our coordinate axes are orthogonal. By this reasoning, we could conjecture that any two obliquely intersecting lines are orthogonal, and then use equations (1) with coordinates based on those lines to confirm that they are indeed orthogonal according to Euclid’s definition. However, the physical objects of our experience would not exhibit spatial isotropy in terms of these coordinates. This illustrates that we can only establish the physical orthogonality of coordinate axes based on physical phenomena. In other words, we construct orthogonal coordinate axes operationally, based on the properties of physical entities. For example, we define an orthogonal system of coordinates in such a way that a certain spatially extended physical entity is isotropic. Then, by definition, this physical entity is isotropic with respect to these coordinates, so again the reasoning is circular. However, the physical significance of these coordinates and the associated spatial isotropy lies in the empirical fact that a large class of physical entities (i.e., “solids”) exhibit spatial isotropy in terms of this same system of coordinates.

 

Next we need to determine a time axis that is orthogonal to each of the space axes. In common words, this amounts to synchronizing the times at spatially separate locations. Just as in the case of the spatial axes, we can establish physically meaningful orthogonality for the time axis only operationally, based on some reference physical phenomena. As we’ve seen, orthogonality between two lines is determined by the distances between points on those lines, so in order to determine a time axis orthogonal to a space axis we need to evaluate “distances” between points that are separated in time as well as in space. Unfortunately, equation (1) defines distances only between points at the same time. Evidently to establish orthogonality between space and time axes we need a physically meaningful measure of space-time distance, rather than merely spatial distance.

 

Another physical symmetry that we observe in nature is the symmetry of temporal translation. This refers to the fact that for a certain class of physical processes the duration of the process is independent of the absolute starting time. In other words, letting t1 and t2 denote the times of the two ends of the process, the quantity

 

 

is invariant under translation of the starting time t1. This is exactly analogous to the symmetry of a class of physical objects under spatial translations. However, we have seen that the spatial symmetries are valid only if the time coordinates t1 and t2 are the same, so we should recognize the possibility that the physical symmetry expressed by the invariance of (2) is valid only when the spatial coordinates of events 1 and 2 are the same. This can only be determined empirically. Somewhat surprisingly, common experience suggests that the values of T2 for a certain class of physical processes actually are invariant even if the spatial positions of events 1 and 2 are different… at least to within the accuracy of common observation and for differences in positions that are not too great. Likewise we find that, for just about any time axis we choose, such that some material object is at rest in terms of the coordinate system, the spatial symmetries indicated by equation (1) apply, at least within the accuracy of common observation and for objects that are not moving too rapidly. This all implies that the ratio of spatial to temporal units of distance is extremely great, if not infinite.

 

If the ratio is infinite, then every time axis is orthogonal to every space axis, whereas if it is finite, any change of the direction of the time axis requires a corresponding change of the spatial axes in order for them to remain mutually perpendicular. The same is true of the relation between the space axes themselves, i.e., if the scale factor between (say) the x and the y coordinates was infinite, then those axes would always be perpendicular, but since it is finite, any rotation of the x axis (about the z axis) requires a corresponding rotation of the y axis in order for them to remain orthogonal. It is perhaps conceivable that the scale factor between space and time could be infinite, but it would be very incongruous, considering that the time axis can have spatial components. Also, taking equations (1) and (2) separately, we have no means of quantifying the absolute separation between two non-simultaneous events. The spatial separation between non-simultaneous events separated by a time increment Δt is totally undefined, because there exist perfectly valid reference frames in which two non-simultaneous events are at precisely the same spatial location, and other frames in which they are arbitrarily far apart. Still, in all of those frames (according to Galilean relativity), the time interval remains Δt. Thus, there is no definite combined spatial and temporal separation – despite the fact that we clearly intuit a definite physical difference between our distance from "the office tomorrow" and our distance from "the Andromeda galaxy tomorrow". Admittedly we could postulate a universal preferred reference frame for the purpose of assessing the complete separations between events, but such a postulate is entirely foreign to the logical structure of Galilean space and time, and has no operational significance.

 

So, we are led to suspect that there is a finite (though perhaps very large) scale factor c between the units of space and time, and that the physical symmetries we’ve been discussing are parts of a larger symmetry, comprehending the spatial symmetries expressed by (1) and the temporal symmetries expressed by (2). On the other hand, we do not expect spacelike intervals and timelike intervals to be directly conformable, because we cannot turn around in time as we can in space. The most natural supposition is that the squared spacelike intervals and the squared timelike intervals have opposite signs, so that they are mutually “imaginary” (in the numerical sense). Hence our proposed invariant quantity for a suitable class of repeatable physical processes extending uniformly from event 1 to event 2 is

 

 

(This is the conventional form for spacelike intervals, whereas the negative of this quantity, denoted by τ2, is used to signify timelike intervals.) This quantity is invariant under any combination of spatial rotations and changes in the state of uniform motion, as well as simple translations of the origin in space and/or time. The algebraic group of all transformations (not counting reflections) that leave this quantity invariant is called the Poincare group, in recognition of the fact that it was first described in Poincare’s famous “Palermo” paper, dated July 1905. Equation (3) is not positive-definite, which means that even though it is a squared quantity it may have a negative value, and it vanishes along the path of a light pulse. Noting that squared times and squared distances have opposite signs, Minkowski remarked that

 

Thus the essence of this postulate may be clothed mathematically in a very pregnant manner in the mystic formula

 

As Poincare had already noted, using an imaginary unit of time allows equation (3) to be re-written in a way that is formally symmetrical in the space and time coordinates, but of course the invariant quantity remains non-positive-definite. The significance of this “mystic formula” continues to be debated, but it does provide an interesting connection to quantum mechanics, to be discussed in Section 9.8.

 

As an aside, note that measurements of physical objects in various orientations are not sufficient to determine the “true” lengths in any metaphysical absolute sense. If all physical objects were, say, twice as long when oriented in one particular absolute direction than in the perpendicular directions, and if this anisotropy affected all physical phenomena equally, we could never detect it, because our rulers would be affected as well. Thus, when we refer to a physical symmetry (such as the isotropy of space), we are referring to the fact that all physical phenomena are affected by some variable (such as spatial orientation) in exactly the same way, not that the phenomena bear any particular relationship with some metaphysical standard. From this perspective we can see that the Lorentzian approach to “explaining” the (apparent) symmetries of space-time does nothing to actually explain those symmetries; it is simply a rationalization of the discrepancy between those empirical symmetries and an a priori metaphysical standard that does not possess those symmetries.

 

In any case, we’ve seen how a slight (for most purposes) modification of the relationship between inertial coordinate systems leads to the invariant quantity

 

 

For any fixed value of the constant c, we will denote by Gc the group of transformations that leave this quantity unchanged. If we let c go to infinity, the temporal increment dt must be invariant, leaving just the original Euclidean group for the spatial increments. Thus the space and time components are de-coupled, in accord with Galilean relativity. Minkowski called this limiting case G∞, and remarked that

 

Since Gc is mathematically much more intelligible than G∞ , it looks as though the thought might have struck some mathematician, fancy-free, that after all, as a matter of fact, natural phenomena do not possess invariance with the group G∞, but rather with the group Gc, with c being finite and determinate, but in ordinary units of measure extremely great.

 

Minkowski is here clearly suggesting that Lorentz invariance might have been deduced from a priori considerations, appealing to mathematical "intelligibility" as a criterion for the laws of nature. Einstein himself eschewed the temptation to retroactively deduce Lorentz invariance from first principles, choosing instead to base his original presentation of special relativity on two empirically-founded principles, the first being none other than the classical principle of relativity, and the second being the proposition that the speed of light is the same with respect to any system of inertial coordinates, independent of the motion of the source. This second principle often strikes people as arbitrary and unwarranted (rather like Euclid's "fifth postulate", as discussed in Section 3.1), and there have been numerous attempts to deduce it from some more fundamental principle. For example, it's been argued that the light speed postulate is actually redundant to the relativity principle itself, since if we regard Maxwell's equations as fundamental laws of physics, and we regard the permeability μ0 and permittivity ε0 of the vacuum as invariant constants of those laws in any uniformly moving frame of reference, then it follows that the speed of light in a vacuum is c =  with respect to every uniformly moving system of coordinates. The problem with this line of reasoning is that Maxwell's equations are not valid when expressed in terms of an arbitrary uniformly moving system of coordinates. In particular, they are not invariant under a Galilean transformation − despite the fact that systems of coordinates related by such a transformation are uniformly moving with respect to each other. (Maxwell himself recognized that the equations of electromagnetism, unlike Newton's equations of mechanics, were not invariant under Galilean "boosts"; in fact he proposed various experiments to exploit this lack of invariance in order to measure the "absolute velocity" of the Earth relative to the luminiferous ether. See Section 3.3 for one example.)

 

Furthermore, we cannot assume, a priori, that μ0 and ε0 are invariant with respect to changes in reference frame. Actually μ0 is an assigned value, but ε0 must be measured, and the usual means of empirically determining ε0 involve observations of the force between charged plates. Maxwell clearly believed these measurements must be made with the apparatus "at rest" with respect to the ether in order to yield the true and isotropic value of ε0. In sections 768 and 769 of Maxwell’s Treatise he discussed the ratio of electrostatic to electromagnetic units, and predicted that two parallel sheets of electric charge, both moving in their own planes in the same direction with velocity c (supposing this to be possible) would exert no net force on each other. If Maxwell imagined himself moving along with these charged plates and observing no force between them, he obviously did not expect the laws of electrostatics to be applicable. (This is analogous to Einstein’s famous thought experiment in which he imagined moving along side a relatively “stationary” pulse of light.) According to Maxwell's conception, if measurements of ε0 are performed with an apparatus traveling at some significant fraction of the speed of light, the results would not only differ from the result at rest, they would also vary depending on the orientation of the plates relative to the direction of the absolute velocity of the apparatus.

 

In retrospect we can see that the efforts of Maxwell and others to devise empirical methods for measuring the absolute rest frame (either by measuring anisotropies in the speed of light or by detecting variations in the electromagnetic properties of the vacuum) were doomed to failure, because even though it's true that the equations of electromagnetism are not invariant under Galilean transformations, it is also true that those equations are invariant with respect to every system of inertial coordinates. Maxwell (along with everyone else before Einstein) would have regarded those two propositions as logically contradictory, because he assumed inertial coordinate systems are related by Galilean transformations. Einstein was the first to recognize that this is not so, i.e., that relatively moving inertial coordinate systems are actually related by Lorentz transformations.

 

Maxwell's equations are suggestive of the invariance of c only because of the added circumstance that we are unable to physically identify any particular frame of reference for the application of those equations. (Needless to say, the same is not true of, for example, the Navier-Stokes equation for a material fluid medium.) The most readily observed instance of this inability to single out a unique reference frame for Maxwell's equations is the empirical invariance of light speed with respect to every inertial system of coordinates, from which we can infer the invariance of ε0. Hence attempts to deduce the invariance of light speed from Maxwell's equations are fundamentally misguided. Furthermore, as discussed in Section 1.6, we know (as did Einstein) that Maxwell's equations are not fundamental, since they don't encompass quantum photo-electric effects (for example), whereas the Minkowski structure of spacetime (representing the invariance of the local characteristic speed of light) evidently is fundamental, even in the context of quantum electrodynamics. This strongly supports Einstein's decision to base his kinematics on the light speed principle itself. (As in the case of Euclid's decision to specify a "fifth postulate" for his theory of geometry, we can only marvel in retrospect at the underlying insight and maturity that this decision reveals.)

 

Another argument that is sometimes advanced in support of the second postulate is based on the notion of causality. If the future is to be determined by (and only by) the past, then (the argument goes) no object or information can move infinitely fast, and from this restriction people have tried to infer the existence of a finite upper bound on speeds, which would then lead to the Lorentz transformations. One problem with this line of reasoning is that it's based on a principle (causality) that is not unambiguously self-evident. Indeed, if certain objects could move infinitely fast, we might expect to find the universe populated with large sets of indistinguishable particles, all of which are really instances of a small number of prototypes moving infinitely fast from place to place, so that they each occupy numerous locations at all times. This may sound implausible until we recall that the universe actually is populated by apparently indistinguishable electrons and protons, and in fact according to quantum mechanics the individual identities of those particles are ambiguous in many circumstances. John Wheeler once seriously toyed with the idea that there is only a single electron in the universe, weaving its way back and forth through time. Admittedly there are problems with such theories, but the point is that causality and the directionality of time are far from being straightforward principles.

 

Moreover, even if we agree to exclude infinite speeds, i.e., that the composition of any two finite speeds must yield a finite speed, we haven't really accomplished anything, because the Galilean composition law has this same property. Every real number is finite, but it does not follow that there must be some finite upper bound on the real numbers. More fundamentally, it's important to recognize that the Minkowski structure of spacetime doesn't, by itself, automatically rule out speeds above the characteristic speed c (nor does it imply temporal asymmetry). Strictly speaking, a separate assumption is required to rule out "tachyons". Thus, we can't really say that Minkowskian spacetime is prima facie any more consistent with causality than is Galilean spacetime.

 

A more persuasive argument for a finite upper bound on speeds can be based on the idea of locality, as mentioned in our review of the shortcomings of the Galilean transformation rule. If the spatial ordering of events is to have any absolute significance, in spite of the fact that distance can be transformed away by motion, it seems that there must be some definite limit on speeds. Also, the continuity and identity of objects from one instant to the next (ignoring the lessons of quantum mechanics) is most intelligible in the context of a unified spacetime manifold with a definite non-singular connection, which implies a finite upper bound on speeds. This is in the spirit of Minkowski's 1908 lecture in which he urged the greater "mathematical intelligibility" of the Lorentzian group as opposed to the Galilean group of transformations.

 

Perhaps the most compelling argument comes from the conservation of energy, which implies that energy is a real palpable entity that can neither be created nor destroyed, but can only move from place to place, subject to dynamical laws. If the bound energy E has inertia equivalent to E/c2, it follows that inertial coordinate systems (i.e., systems in which inertia is homogeneous and isotropic) are related by Lorentz transformations.

 

For a typical axiomatic derivation of the Lorentz transformation, we may begin with the basic Galilean program of seeking to identify coordinate systems with respect to which physical phenomena are optimally simple. We have the fundamental principle that for any material object in any state of motion there exists a system of space and time coordinates with respect to which the object is instantaneously at rest and Newton's laws of inertial motion hold good (at least quasi-statically). Such a system is called an inertial rest frame coordinate system of the object. Let x,t denote inertial rest frame coordinates of one object, and let x′,t′ denote inertial rest frame coordinates of another object moving with a speed v in the positive x direction relative to the x,t coordinates. How are these two coordinate systems related? We can arrange for the origins of the coordinate systems to coincide. Also, since these coordinate systems are defined such that an object in continuous uniform motion with respect to one such system must be in continuous uniform motion with respect to all such systems, and such that inertia is homogeneous and isotropic, it follows that they must be linearly related by the general form x′ = Ax + Bt and t′ = Cx + Dt, where A,B,C,D are constants for a given value of v. The differential form of these equations is dx′ = Adx + Bdt and dt′ = Cdx + Ddt.

 

Now, since the second object is stationary at the origin of the x′,t′ coordinates, it's position is always x′ = 0, so the first transformation equation gives 0 = Adx + Bdt, which implies dx/dt = –B/A = v and hence B = –Av. Also, if we solve the two transformation equations for x and t we get (AD–BC)x = Dx′ – Bt′ and (AD–BC)t = –Cx′ + At′. By reciprocity (relativity and isotropy) the first object is moving with velocity –v relative to the x′,t′ coordinates, so we have –v = dx′/dt′ = B/D, which implies B = –Dv and hence A = D. Furthermore, by isotropy, the transformation and its inverse must not have different scale factors, so the determinant AD – BC = A2 + vAC of the transformation must equal unity, and hence we have C = (1 − A2)/(vA). Combining all these facts, a relativistic and isotropic transformation from one system of inertial coordinates to another must be of the form

 

 

It only remains to determine the value of A (as a function of v), which we can do by fixing the quantity in the square brackets. Letting k denote this quantity for a given v, the transformation can be written in the form

 

 

Any two inertial coordinate systems must be related by a transformation of this form, where v is the mutual speed between them. This implies

 

 

Given three systems of inertial coordinates with the mutual speed v between the first two and u between the second two, the transformation from the first to the third is the composition of transformations with parameters kv and ku. Letting xʺ,tʺ denote the third system of coordinates, we have by direct substitution

 

 

The coefficient of t in the denominator of the right side must be unity, so we have ku = kv, and therefore k is a constant for all v, with units of an inverse squared speed. Also, the coefficient of t in the numerator must be the mutual speed between the first and third coordinate systems. Thus, letting w denote this speed, we have

 

 

With this condition the coefficient of x in the denominator of the composite ratio also takes the required form.

 

Now, if the value of the constant k is non-zero, we can normalize its magnitude by a suitable choice of space and time units, so that the only three fundamentally distinct possibilities to consider are k = −1, 0, and +1. Setting k = 0 gives the familiar Galilean transformation xʹ = x – vt, tʹ = t. This is highly asymmetrical between the time and space parameters, in the sense that it makes the transformed space parameter a function of both the space coordinate and the time coordinate of the original system, whereas the transformed time coordinate is dependent only on the time coordinate of the original system.

 

Alternatively, for the case k = −1 we have the transformation

 

 

Letting θ denote the angle that the line from the origin to the point (x,t) makes with the t axis, then tan(θ) = v = dx/dt, and we have the trigonometric identities cos(θ) = 1/(1+v2)1/2 and sin(θ) = v/(1+v2)1/2. Therefore, this transformation can be written in the form

 

 

which is just a Euclidean rotation in the xt plane. Under this transformation the quantity (dx)2 + (dt)2 = (dx′)2 + (dt′)2 is invariant. This transformation is clearly too symmetrical between x and t, because know from experience that we cannot turn around in time as easily as we can turn around in space.

 

The only remaining alternative is to set k = 1, which gives the transformation

 

 

Although perfectly symmetrical, this maintains the absolute distinction between spatial and temporal intervals. This can be parameterized as a hyperbolic rotation

 

 

and we have the invariant quantity (dx)2 – (dt)2 = (dxʹ)2 – (dtʹ)2 for any given interval. It's hardly surprising that this transformation, rather than either the Galilean transformation or the Euclidean transformation, gives the actual relationship between space and time coordinate systems with respect to which inertia is directionally symmetrical and inertial motion is linear. From purely formal considerations we can see that the Galilean transformation, given by setting k = 0, is incomplete and has no spacetime invariant, whereas the Euclidean transformation, given by setting k = −1, makes no distinction at all between space and time. Only the Lorentzian transformation, given by setting k = 1, has completely satisfactory properties from an abstract point of view, which is presumably why Minkowski referred to it as "more intelligible". Of course, to specify the physical content of the transformation, we must identify the constant k in conventional units. It can be shown that any quantity E of bound energy has inertia kE, so the inertia of energy requires that k = 1/c2 where c is the propagation speed of massless energy.

 

As plausible as such arguments may be, they don't amount to a logical deduction, so some additional fact, beyond the principle of relativity and isotropy, is needed to establish the relationship between inertial coordinate systems. Einstein's light speed postulate can serve this purpose - indeed, in Minkowski’s discussion of “staircase wit” he admited that "the impulse and true motivation for assuming the group Gc came from the fact that the differential equation for the propagation of light in empty space possesses the group Gc", and he referred back to Voigt's 1887 paper (see Section 1.4). More fundamentally, the conservation of energy implies that all forms of energy (including kinetic energy) have inertia, and hence inertial coordinate systems are related by Lorentz transformations.

 

Nevertheless, it's still interesting to explore various rational "intelligibility" arguments that can be put forward as to why space and time must be Minkowskian. One approach is to begin with three speeds u,v,w representing the pairwise speeds between three co-linear particles, and to seek a composition law of the form Q(u,v,w) = 0 relating these speeds. It's easy to make the case that it should uniquely determine any of the speeds in terms of the other two, which implies that Q must be linear in all three of its arguments. The most general linear function of three variables is

 

 

where A,B,...H are constants. Treating the speeds symmetrically requires B = C = D and E = F = G. Also, if any two of the speeds is 0 we require the third speed to be 0 (transitivity), so we have H = 0. Also, if any one of the speeds, say u, is 0, then we require v = -w (reciprocity), but with u = 0 and v = -w the formula reduces to –Dv2 + Fv – Gv = 0, and since F = G (= E) this is just Dv2 = 0, so it follows that B = C = D = 0. Hence the most general function that satisfies our requirements of linearity, 3-way symmetry, transitivity, and reciprocity is Q(u,v,w) = Auvw + E(u+v+w) = 0. It's clear that E must be non-zero (since otherwise general reciprocity would not be imposed when any one of the variables vanished), so we can divide this function by E, and let k denote A/E, to give

 

 

We see that this k is the same as the one discussed previously. As before, the only three distinct cases are k = −1, 0, and +1. If k = 0 we have the Galilean composition law, and if k = 1 we have the Einsteinian composition law. How are we to decide? In the next section we consider the problem from a slightly different perspective, and focus on a unique symmetry that arises only with k = 1.

 

Return to Table of Contents