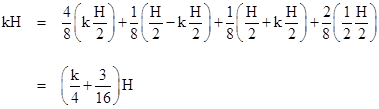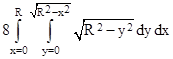Archimedes on Spheres and Cylinders

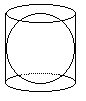
Archimedes was killed during the massacre that followed the capture of Syracuse by the Romans under general Marcellus in 212 BC. Marcellus had supposedly given orders that the renowned sage (who was then 75) was not to be harmed, but (according to one story) in the midst of the general mayhem a Roman soldier came upon an old man drawing pictures in the sand, and ran him through with a sword. Marcellus saw to it that Archimedes was given a burial in accordance with his wishes, including a monument featuring a stone sphere and cylinder. Archimedes regarded his discoveries concerning the volumes and surface areas of these solids as his most important – and most beautiful – achievements. Alas, during the century that followed, this monument to one of history's greatest scientists fell into neglect and was forgotten.

Cicero (106-43 BC), the great Roman statesman and orator, learned as a boy about Archimedes, and later used the mathematician's life as an example of "a good life", in contrast with another Sryacusan, the tyrant Dionysius, who achieved supreme power, but became a prisoner of his own brutal regime and the requirements of maintaining his power. Coincidentally, Cicero's first public office (in 75 BC) was to serve as a quaestor (Roman administrator) in Sicily. In Book V of his "Tusculanarum Disputationum" Cicero digresses in the midst of discoursing on the futility of Dionysius' existence by recounting an interesting episode:

When I was quaestor in Sicily I managed to track down Archimedes' grave. The Syracusans knew nothing about it, and indeed denied that any such thing existed. But there it was, completely surrounded and hidden by bushes of brambles and thorns. I remembered having heard of some simple lines of verse which had been inscribed on his tomb, referring to a sphere and cylinder modelled in stone on top of the grave. And so I took a good look round all the numerous tombs that stand beside the Agrigentine Gate. Finally I noticed a little column, just visible above the scrub: it was surmounted by a sphere and a cylinder. I immediately said to the Syracusans, some of whose leading citizens were with me at the time, that I believed this was the very object I had been looking for. Men were sent in with sickles to clear the site, and when a path to the monument had been opened we walked right up to it. And the verses were still visible, though approximately the second half of each line had been worn away.

So one of the most famous cities in the Greek world, and in former days a great center of learning as well, would have remained in total ignorance of the tomb of the most brilliant citizen it had ever produced, had a man from Arpinum not come and pointed it out! But now to return to the point where I started that digression. Surely anyone who has even the slightest connexion with the Muses, that is to say with civilization and learning, would rather be the mathematician Archimedes than the tyrant Dionysius. If we weigh up the two men's ways of life and behavior, we find that one of them fed his brain on scientific research and discovery, with all the satisfaction that comes with intense intellectual exercise - which is the most wonderful spiritual nourishment in the world - while the thoughts of the other dwelt on murder and oppression, and fear was his companion day and night. Compare Dionysius with Democritus, Pythagoras, Anaxagoras. What thrones or possessions on all the earth could you rank higher than their philosophical achievements, and the satisfaction they derived from them?

That's certainly a fine sentiment regarding the serene contemplation of the universe, untroubled by the baser concerns of life, although it's only fair to recall that both Archimedes and Pythagoras were murdered, and Anaxagoras was imprisoned and sentenced to death for some of his beliefs (e.g., that the Sun was a red-hot stone, and the Moon was made of "earth"). In any case, the tomb of Archimedes subsequently fell into neglect again, and presumably no longer exists.

Why was Archimedes so fond of the sphere and the cylinder? To answer this, we should back up a little, and consider the circle. The number π is defined as the ratio of the circumference to the diameter of a circle, so for any circle we have C = 2πr, by definition. But what is the area of a circle? Is it necessary to define a new number to express this area in terms of the linear dimensions of the circle? Archimedes approached this question very cleverly, realizing that he could easily establish upper and lower bounds on the area of a circle in terms of the circumference and the radius, and then show that those bounds can be made to converge arbitrarily close to each other, leaving only one possibility for the area of a circle.

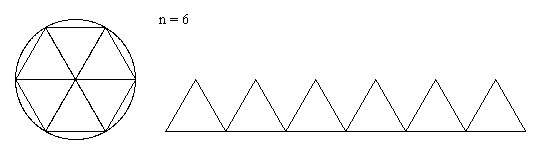
To establish a lower bound on the area of a circle of radius r, suppose we inscribe a regular n-sided polygon within the circle. Notice that this polygon can be decomposed into n identical triangular slices meeting at the center of the circle, as illustrated below for the case n = 6.

The outer edges of these triangles approximate the circumference of the circle. Since a straight line is the shortest path connecting two given points, the sum of these n linear segments is necessarily (slightly) less than the circumference. The "height" of these individual triangles (taking the outer edge as the "base") is slightly less than the radius of the circle. Now, by increasing n, the sum of the outer edges can be made arbitrarily close to the circumference C of the circle, and the "heights" of the triangles can be made arbitrarily close to the radius r. Also, since the area of each triangle is half its "height" times its base, and the sum of their areas is half their height times the sum of their bases, we see that it's possible to inscribe within the circle a regular n-gon whose area is arbitrarily close to rC/2. It follows that the area of the circle cannot be less than rC/2.

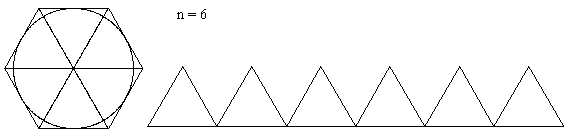
Similarly, by circumscribing a regular n-sided polygon around a circle, as shown below for n = 6, we can easily see that the area of the polygon cannot be greater than rC/2.

Therefore, since the circle's area cannot be either less than or greater than rC/2, it must be rC/2. This is the form of the argument as presented by Archimedes. Of course, since C = 2πr, Archimedes' result is equivalent to the modern formula A = πr² for the area of a circle. This establishes the fact that the number π, which we originally defined as the ratio of the circumference to the diameter of a circle, is also the ratio of the circle's area to the area of a square whose edges equal the circle's radius.

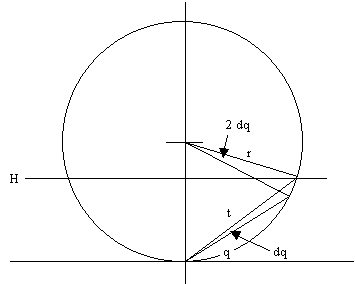
Now let's move on to the sphere. It's natural to ask what is the surface area of a sphere, but Archimedes answered a more general question, giving a recipe for the surface area of any section of a sphere cut off by a plane. Specifically, he discovered and proved that the surface area of a region of a sphere sliced off by a plane equals the area of a circle whose radius is the straight-line distance from the central point of that region to the perimeter. To prove this, consider a sphere cut by a horizontal plane as shown in the side view below.

Archimedes' theorem is that the surface area of the region of the sphere below the horizontal plane H is equal to the area of a circle of radius t. This is obviously true if H is just tangent to the bottom of the sphere, since in that case both areas are zero. Our approach will be to show that an incremental change in the height of H (or, equivalently, in the angle θ) changes the two surface areas by the same amount, so they are always equal.

Since we've already described Archimedes' method of "exhaustion", i.e., his method of deducing results in infinitesimal reasoning by excluding all other possibilities, let's take advantage of some trigonometry and calculus notation to simplify the derivation. Notice that the distance from the end of the vector t to the center of the circle is r, so we have

from which it follows that t = 2r sin(θ). Now, using the formalism of calculus to sketch Archimedes' argument, we can see that dt/dθ = 2r cos(θ), which is to say (informally) that the incremental change dt in t for an incremental change dθ in θ is dt = 2r cos(θ) dθ. We know from our earlier result that the area of a circle of radius t is A = πt², so the incremental change dA in the area A corresponding to an incremental change dt in the length of t is dA = 2πt dt. Multiplying (dt/dθ) and (dA/dt) gives dA/dθ, which can be written as

which tells us how the area of a circle of radius t changes as we change the angle θ. Now, how does the surface area of the sphere below the plane H change for the same change in θ? Well, if the vector t sweeps out an incremental angle dθ, the radial vector r sweeps out an angle 2dθ. (This is a nice fact of elementary trigonometry, as discussed in the note on Loci of Equi-angular Points.) Also, we know that the arc length swept out by an angular displacement 2dθ is equal to 2dθ r, so this is the width of the incremental stripe on the surface of the sphere. The circumference of this stripe is obviously 2πt cos(θ), so the incremental change in surface area of the spherical region is the product of these, which is exactly the same as the incremental change in the area of a circle of radius t. Hence we have proved Archimedes' result.

In particular, suppose we raise the plane H so that it is tangent to the top of the sphere. Archimedes' theorem then tells us that the surface area of the entire sphere equals the area of a circle of radius t = 2r, so we have A_sphere = π(2r)² = 4πr². This is one of the results that Archimedes valued so highly, because it shows that the surface area of a sphere is exactly 4 times the area of a circle with the same radius. Of course, every school child knows this today, but if we imagine a time when this was not known, and then discovering it, we can appreciate that it is a truly beautiful result.

But where is the cylinder in all of this? Notice that the surface area around the circular walls of a cylinder of radius r and height 2r (i.e., a cylinder circumscribed on a sphere) is equal to (2πr)(2r) = 4πr², the same as the surface area of the inscribed sphere. Archimedes showed that not only are these total areas equal, but the areas cut off by any planes perpendicular to the cylinder's axis are also equal. In other words, the surface area of the sphere over any vertical span is equal to the surface area of the circumscribing cylinder over that same span. To see this, let's look again at the side view of the sphere, this time inscribed in a cylinder whose flat faces are tangent to the top and bottom of the sphere, as shown below.

The incremental surface area of the cylinder slice between the planes A and B is 2πr dy, and the surface area of the sphere between those planes is 2πx dc. Also, we see that the right triangle with edge lengths x,y and hypotenuse r is similar to the right triangle with edge lengths dy,dx and hypotenuse dc, so we have dc/dy = r/x, which implies x dc = r dy, and so the surface areas of the sphere and the cylinder between the planes A and B are equal over any vertical span. This is another remarkable result.

Archimedes then went on to consider the volume of the sphere. Before we describe that, let's first recall one of the earliest mathematical theorems concerning geometry, one that seems to have been known (though perhaps only empirically) by the earliest Egyptians and Babylonians, namely, that the volume of a right cone equals 1/3 the volume of the circumscribing cylinder. More generally, the volume of any right pyramid is 1/3 the volume of the prism on the same base with the same height. Archimedes attributed the first clear statement of this fact to Democritus, but said that the first truly scientific proof was due to Eudoxus, who applied his "method of exhaustion".

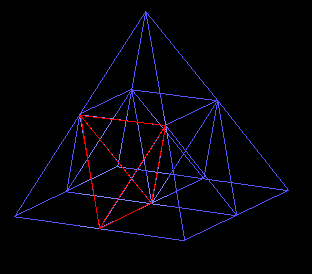
How would we prove this with modern methods? If the area of the base is A, and if we tip this solid upside down, the area on any horizontal slice can be expressed as a function of the height h above the vertex as A(h) = (A/H²)h². Integrating this from h = 0 to h = H gives (1/3)AH, which is indeed 1/3 the volume of the circumscribing prism or cylinder. On the other hand, we can deduce this same result without integration, simply based on the fact that the volumes of similar solids are proportional to the cube of their linear dimension, e.g. the volume of a sphere is proportional to the cube of the radius (which is another result sometimes attributed to Eudoxus). Consider a right pyramid on a square base, and pass a plane through this pyramid parallel to its base at half the height. Then partition this solid as shown below.

We see that the pyramid is comprised of six equal sub-pyramids (one of them upside down), each similar to and half the height of the original, plus four copies of a tetrahedral solid typified by the one outlined in red. Now, we know that the volume of each sub-pyramid is 1/8 the volume of the total pyramid (since the cube of 1/2 is 1/8). Hence these sub-pyramids take up 3/4 of the original pyramid, leaving 1/4 to be taken up by the four identical tetrahedral solids. Therefore, each of those has 1/16 of the total pyramid's volume, which is 1/2 of the volume of a sub-pyramid.

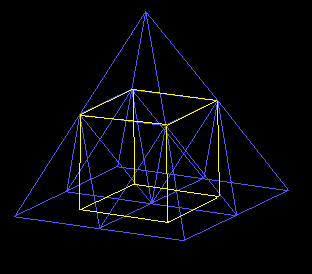
Now drop perpendiculars from the base corners of the upper sub-pyramid to the base of the main pyramid, which gives the prism circumscribing the upside-down sub-pyramid shown in yellow below.

In addition to containing the upside-down sub-pyramid, this prism also contains 1/4 of each of the four lower sub-pyramids, and 1/2 of each of the four tetrahedral solids. Together, these extra pieces represent the volume of two full sub-pyramids, so the entire yellow prism has a volume equal to three times the volume of the inscribed pyramid. Consequently, by similarity, each pyramid's volume is exactly 1/3 the circumscribing prism.

It might seem that this visual approach is only applicable to the special case of a right pyramid on a square base, but we immediately see that if we stand such a pyramid next to any prism and pyramid, or cylinder and cone, of the same height, and pass horizontal planes through both solids parallel to the common plane of their bases, then the areas of the circumscribing and interior solids, respectively, are always in constant proportion, so this suffices to prove that any linearly tapering pointed solid on an arbitrary base has 1/3 the volume of the prism on the same base with the same height.

Incidentally, this same partition of the pyramid enables us to determine that the average height of the points of this solid is exactly 1/4 the height of the pyramid itself. If the average height of the points of a pyramid of height H is kH for some constant k, and noting that the average height of a tetrahedron is H/2, we have

from which we get k = 1/4. (This is another example of the kind of technique discussed in the note Why Calculus?.)

Finally, let's consider the volume of the sphere. Once again Archimedes found that we can express this quantity as a simple rational multiple of the number π. The area of the circular slice of a sphere of radius R cut by a plane at a distance y above the equatorial plane is A = πx² where x² + y² = R². Hence it's trivial using modern integration to compute the volume of the sphere as follows

Since the volume of the circumscribing cylinder is obviously 2πR³, we have Archimedes' result that the sphere has 2/3 the volume of the circumscribing cylinder. Interestingly, if we include the two flat ends of the cylinder, it's surface area is 6πR², so the sphere's surface area is 2/3 the total surface area of the circumscribing cylinder. This delighted Archimedes, to have shown that both the volume and the surface area of a sphere is 2/3 that of the circumscribing cylinder.

To conclude, it's interesting to see how the ingenious methods of Archimedes can sometimes gives results that bypass some fairly elaborate calculus. To illustrate, consider how one would determine the volume removed from a steel cylinder of radius R when a round hole of radius R is drilled through it, perpendicular to the cylinder's length. It's important that the hole's diameter is the same as the cylinder's, because otherwise the answer is an elliptic integral that usually won't be expressible in any "nice" form. Now, given that the radius of the cylinder and the radius of the hole are both R, we can cut the cylinder in half by a plane containing its axis, and then set up orthogonal coordinates x,y in the plane and z normal to the plane. Drill the hole along the z axis through the origin. The volume removed is just (twice) the integral of the cylinder's height above the plane, evaluated over the hole's circular "footprint" on the plane. We really only need to evaluate 1 quadrant of the footprint, because they are symmetrical. Thus, the answer is given by the double integral

which happens to work out nicely to (16/3)R³. But there's a more clever way of solving this problem. Imagine two cylinders of equal radius intersecting each other at right angles. Our problem is to find the volume of intersection. Now imagine a sphere inscribed inside both cylinders, so it is inside the intersection. If we slice off along a plane surface parallel to both of the cylinder axes, the intersection of the cylinders will be represented by a square region on this plane, and the sphere will be a circular region. Also, it's not hard to see that the circular region will always be inscribed in the square region, i.e., the edges of the square will be tangent to the circle (because the sphere touches each cylinder at every "height"). Therefore, at every height the slice of area in the cylinder intersection is 4/π times the area of the slice of the sphere, so the total volume of the region of intersection is 4/π times the volume of the sphere, which is (4/π)(4/3)π R³ = (16/3)R³, in agreement with what we found previously.

Return to MathPages Main Menu