|
Guillaume, Guillaume, and Einstein |
|
|
|
During the search for this slight refinement, an unsuspected anomaly came to light, which was a direct manifestation of the struggle between the various states of iron to which the association of nickel gives an unexpected shape, creating a class of alloys whose properties, unique so far, satisfy in a quite unhoped-for manner the wishes which metrologists would have formulated, had it not been assumed that such properties could not exist in metals. |
|
Charles Edouard Guillaume, 1920 |
|
|
|
|
|
|
|
Charles Edouard Guillaume (1861-1936) attended the Ecole Polytechnique in Bern and after getting his doctorate in physics and serving his obligatory term in the Swiss army he took a job with the International Bureau of Weights and Measures in Paris, where he continued to work for the next 56 years, until his death in 1936. His younger cousin, Edouard Giullaume (1881-1959), also got a doctorate in physics, and worked as a patent examiner at the Swiss patent office in Bern. It was there that Edouard met Albert Einstein (1879-1955), another graduate of the Polytechnique, who worked as a patent examiner in the same office from 1902 until gaining his first professorship in 1909. |
|
|
|
There is no record of the personal relationship between Edouard Guillaume and Einstein while both were patent examiners in Bern, but they were surely acquainted with each other. During the years between 1905 and 1909 Einstein gained international renown based on his remarkable papers on the theory of specific heats, Brownian motion, the photoelectric effect, and especially relativity. The latter subject seems to have rankled Guillaume, and beginning in 1913 (after Einstein had left the patent office to become a professor, first at Zurich and then Prague), he published a series of papers in the Archives des Sciences Physiques et Naturelles in which he argued for a Lorentzian electrodynamics that retained the concept of a universal time. This was directly contrary to Einstein’s relativistic interpretation, which Guillaume consistently deprecated. As Einstein’s fame grew and the “theory of relativity” gained acceptance, Guillaume become more and more determined to convince the scientific community that it was misguided. He announced at one scientific gathering that “I will destroy relativity”. |
|
|
|
Einstein initially disregarded Guillaume’s attacks on special relativity, which is understandable, considering that he (Einstein) was intensely occupied during these years with the search for a more general theory to encompass gravitation. However, in 1917, after completing the general theory, he finally took notice of Guillaume, prompted by receipt from the Swiss Society of Physics of a reprinting of one of Guillaume’s papers on his interpretation of the Lorentz transformation. In a private letter to Guillaume on 24 September 1917 Einstein wrote |
|
|
|
This interpretation is impossible, however, because the three equations [in Guillaume’s paper] cannot be reconciled with one another. The “t” you introduce does not exist if the Lorentz transformation is retained. I shall not return to the matter publicly if you do not force me to by emphasizing it constantly. |
|
|
|
(This and all subsequent excerpts from Einstein’s correspondence are taken from The Collected Papers of Albert Einstein, published by Princeton University Press.) Guillaume had been trying to attract Einstein’s attention for years, and on October 3 he immediately replied |
|
|
|
Finally I get something from you! First I wrote you, then I sent you my papers (admittedly to your former address), without getting any sign of life from you. I think a misunderstanding exists. The transformation expressed in homogeneous coordinates… can be regarded in purely formal terms as a normal projective relationship between 2 point systems. If the coordinates are then derived with reference to a parameter t, rules are obtained for the “addition” of the “velocities”… which permit the classical optical experiments, etc., without admitting the “contraction”, and this without any inner inconsistency. That is all. I would be very grateful if you would reply to me at length, and it would be my pleasure to have your refutation appear in the Archives. |
|
|
|
To this, on October 9, Einstein promptly answered back, with emphasis |
|
|
|
That parameter t just does not exist. The equation u′ = β(u – αx) excludes both of the equations u = ct and u′ = c′t, from which, and in contradiction to the [former] equation, the equation u′ = const ∙ u would indeed result. Should I really let this be printed in the Archives? |
|
|
|
In a letter written on October 17, the now agitated Guillaume continued to press his case: |
|
|
|
When integrating, the integration constants do not have to be omitted! The equations du/dt = c and du′/dt = c′ upon integration result in u = ct + r and u′ = c′t + r′, where, although r and r′ are independent of t, they are nevertheless dependent on x or x′. Later I shall give you a complete report about this new “kinematic theory of relations”, since my current papers do not contain any sufficient explanation of it. |
|
|
|
Since Guillaume had been publishing on this subject for several years, and his comments really amount to nothing but high school algebra, it seems hard to understand why none of his papers “contain any sufficient explanation of it”. Anyone who has ever received notification of an unintelligible ‘new theory of physics’ of this kind will immediately recognize the common attributes of such communications in Guillaume’s letters. Once again Einstein replied from Berlin |
|
|
|
In my opinion this new point of view is also untenable. If “t” is supposed to be a function of u and x, it must be possible to specify this function explicitly. Upon calm consideration, you will yourself become convinced of the non-existence of a “t” to which the role of a universal time could be ascribed. |
|
|
|
It’s interesting that Einstein should take this line, since he readily conceded in correspondence with Lorentz that special relativity admits of an absolutist interpretation with a universal time, albeit one that Einstein regarded as pointless. In any case, although it’s hard to imagine that Guillaume would have been satisfied with Einstein’s response, the correspondence seems to have lapsed at this point. |
|
|
|
Two years later, in November of 1919 came the spectacular announcement at the Royal Society in London that the eclipse expeditions had apparently confirmed Einstein’s predicted gravitational bending of the rays of starlight passing near the Sun. Literally overnight he became one of the most famous and celebrated men in the world. This seems to have re-animated Guillaume, because just weeks later, on 25 January 1920, he wrote again to Einstein: |
|
|
|
I do not need to tell you what pleasure the experimental confirmation of your fine theory gave me. That I value your theory very highly already arises from the fact that I have been working intensely on it for two years. Now however I have arrived at results that are so important for the development of the theory that I take it as my duty to inform you of them without delay. A detailed paper will soon appear in the Archives. For my studies of space and time led me to the conclusion that a basic error regarding time is hidden in the theory… |
|
|
|
Following this is a long series of comments that are, as far as I can tell, complete nonsense. Guillaume writes several things that look superficially like mathematical equations, but that don’t actually connect to any physical content. His basic assertion was that the proper time intervals along different worldlines are actually just the same intervals expressed in different units, but his justification for this claim is (to me) unintelligible. Shortly thereafter, on Feb 5, Einstein received a letter from his old friend Marcel Grossmann (who had helped Einstein get his job in the Patent Office back in 1902), in which Grossmann wrote “At Guillaume’s request I forward to you his handwritten and printed notes”. On February 9, Einstein replied to Guillaume |
|
|
|
I was delighted to receive your letter, because there is always a certain pleasure attached to reviving older reminiscences by us ancient fellows. But your observations are definitely a bit foul and are based on an insufficiently sharp distinction between the cases to which the presented equations refer… |
|
|
|
He goes on to describe the two different situations (that Guillaume is treating as the same situation), and explains how special relativity correctly predicts the outcomes in both cases. Einstein concludes by saying |
|
|
|
And now you assert, you rascal (which is what you always were) that these two results are in contradiction with each other! But two entirely different problems are involved, and the only thing either problem has in common is that a quantity, which you designate as ν, appears. |
|
|
|
Predictably (to anyone who has ever been involved in a correspondence of this kind), Guillaume totally failed to grasp Einstein’s explanation, and immediately replied on February 15 |
|
|
|
I am very glad to find that you have kept your good humor despite the difficult times. But you shouldn’t make it so easy for the rascal to reply! |
|
|
|
Guillaume then launched into another round of verbiage and equations, again purporting to prove that all lapses of proper time between two given events are physically the same, and that Einstein’s theory mistakes changes in the units of measurement with physical changes. If the case were described more coherently, this could be taken for the Lorentzian absolutist interpretation, except that Guillaume wants to infer that the relativistic consequences are thereby eliminated, which of course Lorentz knew was not true. Guillaume concludes the letter by proudly pointing out a misprint on page 197 of Weyl’s book Space, Time, Matter, where the word “frequency” appears in place of “period”, an observation that has nothing to do with the subject of the letter. |
|
|
|
Throughout the correspondence, Einstein remained respectful, but his real attitude can be inferred from a letter to Grossmann on February 27, in which he commented on another of Guillaume’s notes published in the Archives: |
|
|
|
Guillaume’s notice is stupid, like everything this man dashes off about relativity. |
|
|
|
If Einstein thought this would not get back to Guillaume, he was mistaken, because on June 20 Guillaume wrote to him again, saying |
|
|
|
I gather you are in possession of my latest paper, and that you have read it – which is probably not the case! As various people have explained to me, however, it would be very fruitful if you could substantiate your position sometime; and for me personally it has become absolutely necessary that you speak up. For in a controversy in the press, your friend Grossmann wrote that my “views about relativity had been completely repudiated (?) by you”. When I thereupon asked Grossmann when and where you had expressed yourself in this way, he replied to me that, in a letter, you had written that my “views on relativity were nonsense”. This groundless judgment, which is apt to hurt me to the highest degree, forces me to insist that either it be given a basis or retracted. That is why I must ask you, dear Einstein, for a reply that I can publicize. This you really can’t refuse your old Office colleague! |
|
|
|
Guillaume then goes on to give yet another “brief summary” of his “interpretation”. This includes the assertion that certain quantities usually regarded as periods are actually “masses of the same time span”. He then gives what seems to be an attempt to describe something like the Heaviside ellipsoid (although he doesn’t call it that), stating that “a light sphere appears, judged from [a relatively moving system of coordinates] as a rotational ellipsoid with one focus at the origin”. It is actually true that, in the Lorentzian interpretation, a light sphere maps to such an ellipsoid (cf. the notes on Corresponding States and Conventional Wisdom), although Guillaume’s description is not clear enough to allow us to say for sure that this is what he was talking about. Also, the conclusion he draws from it, i.e., that Einstein’s theory of relativity is untenable, is obviously erroneous. (Note that Lorentz himself never claimed his ether-based interpretation invalidated Einstein’s interpretation, but merely that both interpretations are viable.) On July 4, Einstein replied to his “old Office colleague” |
|
|
|
My behavior must appear unkind and unloyal to you; it may seem that I wanted to condemn you by my silence. In reality, though, it is entirely otherwise. I often tried to understand your statements but always utterly failed. So if I wrote Grossmann that it was nonsense, this must be understood with reference to me, or better yet, to the present state of my brain; nonsense is what one calls whatever one cannot grasp; there is no other criterion. Now you must excuse me all the more, since papers and manuscripts are raining down on me like whip lashes on a cab horse. But since you attack me so energetically, I have no choice but to try to find elucidation through repeated correspondence… You say θ and θ′ were periods of clocks – hence things, not numbers – and write θτ = θ′τ′. Are these somehow symbolic equations? I cannot comprehend it as an equation between numbers. This is why I cannot understand all the follows, and think that you have temporarily fallen for a forbidden mysticism, in that you forgot that equations deal with numbers… |
|
|
|
Apparently Guillaume sent at least one more letter (which has not survived) by July 14, because Einstein sent another reply on July 19, but he was still unable to make sense of Guillaume’s assertions – even despite the fact that Guillaume claimed Hadamard and Levi-Civita understood him. Einstein wrote: |
|
|
|
I do not understand what you conceive as a “period” in your letter; with me it is not a number but the designation for a cyclic process that repeats itself. Your deduction of the relation θt = θ′t′ seems to me, quite frankly, completely crazy… It makes absolutely no reasonable sense to compare the period length θ with the arrival time t of that plane moving with the velocity of light… In any case, I must emphasize that it seems absolutely senseless to establish a relationship between the quantities θ and t appearing in your consideration. Upon closer reflection you will also find it so. If Hadamard and Levi-Civita are capable of attaching any reasonable sense to this relation (θt = θ′t′), then I cannot understand them; if anything, only envy them. |
|
|
|
He concluded the letter with an amusing postscript: |
|
|
|
You are going to think: “Einstein has become a loutish Boche”. All the more amicable are my greetings to you, yours, A. Einstein |
|
|
|
Repent, you hardened sinner! |
|
|
|
After this letter, Guillaume could have no more illusions as to how Einstein regarded him and his ideas. At this point Einstein’s old friend Michele Besso, another old colleague from the Swiss Patent office, was drawn into the fray. Apparently in a letter that hasn’t survived, Besso must have written that he could see some merit in Guillaume’s writings – or at least this was Einstein’s impression. On July 26 Einstein wrote to his faithful friend Besso a letter in which he vents some of his annoyance. |
|
|
|
He [Guillaume] doggedly keeps writing the same rubbish, according to Napoleon's maxim that repetition is the most effective argument. Despite all the effort I take, I cannot find any sense behind his words and am (for my part) certain that there is none, either. He seems not to be able to distinguish between things and numbers in his considerations. |
|
|
|
He then enters into a lengthy discussion of other scientific matters, leading to Weyl’s brilliant but (in Einstein’s view) misguided “gauge theory” combining electromagnetism and gravity, about which he says |
|
|
|
I was convinced of the inaccuracy (that is, the inapplicability) of Weyl's theory from the very start. Actually, there are almost exclusively factual reasons against it, but not for it. But as long as you believe in [Guillaume], it would be unfair to complain about Weyl, because these are so completely different orders of magnitude of sins; Weyl is always a profound, clear mind, sheer bliss to read, the other, however, is slovenly. |
|
|
|
It almost seems as if by this time Einstein had difficulty bringing himself to write Guillaume’s name, always using various pronouns instead. The rest of Einstein’s letter spoke about the distinction between relative and absolute quantities in physics, and about Einstein’s objections to Weyl’s theory. (Did either of them notice the odd coincidence that both Weyl and Guillaume were proposing “gauge theories”, albeit of “completely different orders of magnitude”?) At the end of the letter Einstein included some personal advice for Besso’s son Vero: |
|
|
|
I would wish for him some practical occupation as soon as possible, something like what we had at the Patent Office, so that he is always put before small, well-defined tasks. |
|
|
|
Besso answered on July 29: |
|
|
|
About G and W’s relative and absolute merits, I am in full agreement. I did not mean that from his letter you would see that it had any sensible meaning, but rather how he arrived at his tragicomical whim- e.g., by a printing error or slip of the pen (as with Weyl, p. 211, frequency appears instead of period, so also with you, some error may have been left standing somewhere). |
|
|
|
Another missing letter from Guillaume prompted yet another reply from Einstein, dated July 31. He says he has succeeded in understanding the first part of the letter, and shows how he would re-write it to make it more clear. Then he says |
|
|
|
If you had defined θ this way earlier, I would have understood it immediately. It is strange and suspicious that you introduce many clocks at once into the consideration that all are supposed to serve for the measurement of time. But no one can forbid you from doing so. I [still] cannot understand the rest of the letter, because I absolutely do not know what you intend by it. All my efforts were futile. I pondered for about two hours. Then I took up your letter of July 14 again, but I could do no better… When you write back to me, please remain consistent in having the θ’s be lengths of a clock’s period measured by a special standard clock. |
|
|
|
Still another round of letters (of which Guillaume’s is missing) followed, with Einstein writing on August 22 that |
|
|
|
…the time differences [referred to by Guillaume] are not measurable by one clock (neither of the two are), rather only by means of a system of clocks that is synchronized with reference to K and is at rest relative to K or, respectively, a system of clocks that is synchronized with reference to K′ and is at rest relative to K′. I absolutely cannot grasp the consideration that you say I would make. I absolutely do not see what I should understand by the frequencies ν and ν′. Consequently I also cannot attach any sense to the [other] relations either… Therefore, none of the rest of your letter is comprehensible to me. Discuss the matter with Besso sometime. Maybe he can act as interpreter since he knows the relevant recesses of my brain inside out. |
|
|
|
Interestingly, just two days later, on August 24, 1920, the “Working Group of German Scientists for the Preservation of Pure Science” held a public meeting in the largest of Berlin’s concert halls, with the expressed purpose of debunking relativity and discrediting Einstein. Some idea of the tenor of this meeting can be gathered from a letter sent by the organizer to Felix Ehrenhaft inviting him to speak at the affair: |
|
|
|
Esteemed Professor, Now that unanimous agreement has been reached among serious members of the exact sciences about rejecting Einstein’s research, we are planning also to present the educated lay public with counter-arguments, after it has long enough been fed to the point of vomiting with Einstein’s ideas… |
|
|
|
Among the mostly undistinguished members of this organization was the Nobel prize-winner Philip Lenard. Of course, by 1920, Einstein himself had been nominated many times for the Nobel prize, but had not received it, largely because many members of the conservative Nobel committee regarded relativity with suspicion if not outright disdain. The monetary award associated with the prize would certainly have been helpful to Einstein, as it had been promised to his ex-wife in their divorce settlement, and would have relieved him of worries about providing for his two sons. Still, despite being nominated repeatedly by other physicists (including Planck, Lorentz, Wein, Bohr, and so on) referring to him as “among the first rank of physicists of all time”, Einstein had not been awarded the prize. |
|
|
|
Einstein attended the well-publicized event at the Berlin Philharmonic on the 24th, and three days later he sent a letter to the editor of the Berlin Tageblatt. It was entitled “My Answer to the Anti-Relativity Theory Company Ltd”, and it clearly expressed his anger at having his reputation and ideas publicly attacked in this way. His friends were appalled, first by the public attack on Einstein, but almost more so by Einstein’s reply. His friend Paul Ehrenfest wrote that he could not believe Einstein would stoop to respond to such rabble, and that he could not believe Einstein had actually written the letter. Is it possible that some of the anger of the letter was due to his frustrations with the on-going correspondence with Guillaume? After all, the arguments of the Anti-Relativity Company were essentially identical to those that Guillaume advocated. Regardless of the provocation, Einstein soon regretted the letter to the newspaper, admitting to his friend Max Born that it had been a mistake, but then saying with a shrug, “everyone has to sacrifice at the altar of stupidity from time to time”. |
|
|
|
In any case, the correspondence with Guillaume continued unabated throughout this episode. On September 4th Einstein wrote again to his old Office colleague, with surprising equanimity, considering the events that were taking place. |
|
|
|
Dear Guillaume, The conclusion about the rate of the moving clock cannot be derived from the formula [proposed by Guillaume]. It rather results directly from the inverse Lorentz transformation… I see from this statement of yours that you have still not fully grasped the special theory of relativity… a single clock can only measure time at a single location… In time evaluations in which many locations (with reference to the coordinate system) are involved, a system of set clocks is always necessary. |
|
|
|
On September 9th, Einstein heard once again from his friend Grossmann. |
|
|
|
In the same post I am sending you a paper by Mr. Charles Willigens from the Archives des sciences physiques et naturelles. As you see, a cult is forming around Guillaume that thinks it must correct essential points of your concepts. Although this matter is unlikely to be of interest to you, I think it would be in the interest of relativity theory if you had a brief joust with Guillaume someday, such as in a short article for the Archives, for which I would gladly provide the translation, or simply in a letter to me, the scientific gist of which I could pass on, which would please our sympathetic colleague Guye very much. There is a danger that from the unchallenged appearances by Guillaume and his disciples—also in the dailies—dissemination of the fundamental ideas of the theory of relativity would suffer harm in the French-speaking region, which is always ready to claim French superiority on this issue as well. All the more so since the depraved campaign against you in Germany is also echoed here. Thus I think I may ask you to let me know in brief outline for what reasons you reject Guillaume's ideas! |
|
|
|
Incidentally, Grossmann always used the salutation “Dear Albert”, whereas Einstein always used “Dear Grossmann”. In his reply on September 12, Einstein wrote: |
|
|
|
This world is a strange madhouse. Currently, every coachman and every waiter is debating whether relativity theory is correct. Belief in this matter depends on political party affiliation. Most amusing, though, is the Guillaumiade [Guillaume contest]. For in it, someone using scientific jargon has been serving the most pitiful nonsense to the illustrious experts in the field for years on end, and this with impunity, without being reprimanded. Thus one sees quite clearly how the judgments and values prevailing among the flock of scholarly sheep rest on the narrow foundation of a few discerning minds. Refutation is not such an easy matter, though, when one is not even in a position to understand the other's assertions. I took every trouble: I thought about it, corresponded with Guillaume for a long time, but met with nothing but mathematical symbols devoid of any sense. A factual sparring is absolutely unthinkable; rather, one can only state an opinion. I enclose one for the Archives with this letter. |
|
|
|
Following this, Einstein discussed the possibility of his leaving Berlin for a position (again) in Zurich (doubtful), and then on a personal note he mentioned that he was pleased his son and Grossmann’s son were now school mates “as we were”. At the conclusion of the letter, Einstein appended his statement on the Guillaume affair. |
|
|
|
For the Archives |
|
In the past few years Mr. E. Guillaume has repeatedly stated his position about the theory of relativity in this journal and, specifically, attempted to introduce a new concept (universal time) into this theory. At the repeated prompting of the author himself as well as of other colleagues in the field, I consider it necessary to declare the following: Despite taking the [greatest] trouble, I have not been able to attach any kind of clear sense to Guillaume's explications. Even by a lengthy exchange of correspondence conducted with utmost patience, I could come no closer to this goal. In particular, it has remained completely unclear to me what the author means by "universal time." My ability to understand does not even go far enough to be capable of a substantive rebuttal. I can only state my conviction that no clear chain of reasoning underlies Guillaume's explications. |
|
|
|
Dear Grossmann, please ask the Archives to send the proofs to Guillaume. The statement is hard, but I can find no other way; this nonsense has gone too far! |
|
|
|
On November 20 Grossmann replied |
|
|
|
I received your letter about Guillaume's work and forwarded it at the time, and know from Prof. Guye, whom I met recently, that your notice is going to appear soon in the Archives. I, for my part, added a short math statement that attempts to grasp the math nonsense, as it is very much more overt than the physics, where everything is obscure shades of gray. |
|
|
|
It’s my understanding that Einstein’s statement was never actually published. Grossmann replaced Einstein’s words with a watered-down version, merely expressing the fact that Einstein did not agree with Guillaume’s ideas on relativity. It’s interesting that, for this little note, the collaboration of Einstein and Grossmann repeated itself, and just as in the case of their famous “Entwurf” paper of 1913, Grossmann confined himself to the mathematics and left the physics (“where everything is obscure shades of gray”) to Einstein. |
|
|
|
But this wasn’t yet the end of the “Guillaume contest”. Guillaume wrote again to Einstein, this time posing some kind of challenge – presumably to a debate in the pages of the Archives. Einstein replied on December 16 |
|
|
|
I have so much obligatory work to do at present that I cannot think of writing a longer paper. Thus I am unfortunately not in a position to accept the friendly challenge. You might write Mr. Xavier Leon that he could address himself to Langevin, who is an outstanding expert in the theory. |
|
|
|
Then he revealed the content of the Grossmann correspondence: |
|
|
|
Grossmann recently asked me for an assessment of your papers in the area of relativity theory because it was supposedly necessary to take an official position on it, finally. I asserted that despite diligent attempts I was unable to make any progress toward comprehension and that I personally was convinced that there is no clear theoretical idea behind it. Don't be cross with me; it was no longer appropriate to keep silent about my opinion on this point. It is impossible to assign, in any meaningful way, a universal time to the totality of inertial systems. |
|
|
|
However, even this was not quite the end. Guillaume sent another letter to Einstein, on December 23. I don’t have access to this letter (it was not selected for translation into English by the Einstein Papers project), but on December 29 there is a letter from Einstein, who apparently has finally been able to decipher Guillaume’s “baseless explications”. |
|
|
|
Now I think I see what you are doing. You are observing a spherical wave. Observed from K1, the world points taken at a time u1 are characterized by a time u1 = const. Observed from K2 these worldpoints lie on a surface that is characterized by the equation |
|
|
|
With reference to K2 alone, however, this surface has absolutely no physical meaning. I know we are dealing with some sort of idée fixe of yours and that all labors of love are futile. I am no angrier at you than at a sparrow for not singing like a nightingale. But it amuses me that you are apparently finding a faithful audience… but certainly no theoretical physicist of any standing will fall for this business of yours. With Langevin, for instance, I am firmly persuaded that he will immediately see the whole picture. I cannot imagine that Grossmann would have anything against you. But he is probably embarrassed for Swiss physicists that the issue is not being challenged; one can’t take offense at that. When asked, I cannot do otherwise than speak my mind. And I don’t know what else to say to you than: do what you just cannot refrain from doing. |
|
|
|
It’s interesting to compare these remarks to the comments Einstein made in a letter to Besso on December 4, 1918 regarding Weyl’s devotion to what he (Einstein) regarded as an untenable theory. Einstein had the greatest respect for Weyl, and for the conceptual beauty of his “gauge invariance” theory, but nevertheless he wrote |
|
|
|
I am firmly convinced that Weyl’s gauge invariance does not apply to nature, and recently imparted to him support for the objections I have. But I know that someone who has stayed infatuated with one idea for more than half a year can no longer be saved from its spell, at least not by others. |
|
|
|
It should be noted that Weyl did, before too long, agree that his theory was not tenable, whereas Guillaume persisted to the end of his life attempting to convince people of the unsoundness of Einstein’s relativity. In addition, Guillaume championed the cause of Poincare as the true originator of special relativity. In this respect Guillaume was similar to most other critics of Einstein’s relativity, from the Anti-Relativity Company Ltd. in 1920 all the way to the present day. The two-fold argument is basically that (1) relativity is completely and self-evidently wrong, and (2) even if relativity is right, it was plagiarized from someone else. (This is somehow reminiscent of the joke about a lawyer who says “Your honor, we will prove, first that my client was never in possession of the plaintiff’s car, second that he returned it in perfect condition, and third that it was already dented when he borrowed it.”) |
|
|
|
But what of the Nobel prize? With most of the world’s most respected physicists nominating Einstein in the strongest terms, did he finally get the award in 1920? No, he did not. In a move that surprised everyone, the Nobel committee decided to give the physics award in 1920 to an individual who had received just a single nomination. Imagine Einstein’s reaction when he learned that the award had been given to none other than Charles Edouard Guillaume, the cousin of his old Patent office colleague and erstwhile correspondent! Reportedly, Guillaume was as surprised as everyone else. |
|
|
|
The story of how Einstein did not get the Nobel prize in 1920, or in 1921, and the famous reports of Arrhenius and Gullstrand downplaying or disparaging relativity, has been much discussed in the literature, but I’ve never seen any discussion of the (admittedly indirect) connection between Einstein and Guillaume, the man who was awarded the 1920 prize. Gullstrand (a member of the Nobel committee) was quoted as saying that “Einstein must never win the Nobel prize for relativity!”, because he was strongly opposed to what he regarded as the overly abstract and mathematical approach to physics. Both Arrhenius and Gullstrand were obviously acquainted with the relativity literature, especially the literature critical of the theory, so it seems plausible that they were familiar with the “other” Guillaume’s battle with Einstein. Is it purely coincidental that the prize, so pointedly denied to Einstein for relativity theory, was awarded (more or less out of the blue) to the cousin of one of the most vocal critics of relativity theory? |
|
|
|
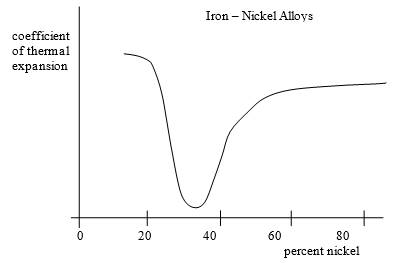
The work for which Charles Edouard Guillaume was cited by the committee was his discovery (in 1896) of the alloy that Guillaume named “Invar”, because it is virtually invariant under changes in temperature. In other words, its coefficient of thermal expansion is virtually zero. He discovered this while searching for a thermally stable material to be used in making precision standard reference equipment and measuring devices for the Bureau of Weights and Measures. Nickel had long been combined with iron in various proportions to produce useful materials. Guillaume varied these proportions to see if he could identify the mixture with the lowest coefficient of thermal expansion. He found that he could make a slightly less temperature-sensitive alloy by adjusting the nickel content over a certain range, but then to his amazement, when he tested the case of 36% nickel and 64% iron, he found that the material’s coefficient of thermal expansion had dropped virtually to zero. A plot of the expansion coefficient versus percent nickel content is shown below. |
|
|
|
|
|
|
|
The generic name for Invar is FeNi36, and it’s average coefficient of expansion is about (0.8)10–6 per degree C, compared with (11)10–6 per degree C for steel. By adding trace amounts of other materials, it is possible to lower to coefficient to zero, or even make it negative. Of course, anomalous coefficients of thermal expansion were not a new phenomenon, since one of the most common every-day substances (H2O) possesses such an anomaly, i.e., water near its freezing point actually has a negative coefficient of expansion, which is why ice floats and why water freezes at the top of a lake rather than at the bottom. However, the existence of such an anomaly in metals was not expected on the basis of any theory of materials. It was as much a surprise as the discovery in the 1990‘s of high-temperature superconductors. In fact, to this day the precise mechanism for Invar’s anomalous behavior is (as recently as 2001) the subject of debate and research. The best current explanation is that it involves the phenomenon of ferromagnetism, but a detailed theoretical explanation has yet to be found. As one manufacturer puts it on their web site, “Over one hundred years after its discovery, Invar is still exhibiting the ‘Invar Effect’ that defies understanding”. |
|
|
|
Guillaume went on to discover a material he named Elinvar, which is characterized by a nearly invariant Young’s modulus (spring coefficient), so it could be used to make watches that run accurately over a range of temperatures. These two materials (Invar and Elinvar) have found uses in a wide range of applications, many of which we take for granted, but which wouldn’t be possible were it not for these special materials. Components of everything from wrist-watches to gas turbine engines have made critical use of Invar and Elinvar. To cite one commonplace example, the metal threads at the base of an ordinary household light bulb would hardly be possible were it not for the existence of a metal whose coefficient of expansion is low enough to remain attached to the glass bulb even when heated. Invar continues to find new uses, in such devices as integrated circuit chips, lasers, communications satellites, tooling for composite aircraft, and many kinds of precision scientific equipment. |
|
|
|
In 1921, the year after Guillaume was awarded the Nobel prize, Einstein was again nominated by many of the world’s leading physicists. Eddington wrote to the committee that “Einstein stands above his contemporaries even as Newton did”. But this is the year in which Gullstrand issued his disparaging report, and the committee decided not to award a physics prize for 1921. The following year, however, the tide had turned. One nominator (Brillouin) asked the committee to “imagine for a moment what the general opinion will be fifty years from now if the name Einstein does not appear on the list of Nobel laureates”. So, in 1922, Einstein was retro-actively awarded the 1921 prize (and Bohr got the 1922 prize). Nevertheless, relativity theory was still seen as too controversial – and too unconnected to the practical world – to be the subject of a Nobel prize. Instead, the committee cited Einstein’s “services to theoretical physics and especially his discovery of the law of the photoelectric effect”. It’s worth noting that the award was not for the theory of light quanta, but rather for discovering the “law” of the photoelectric effect. This again reflects the committee’s reluctance to endorse theoretical ideas. Years later when Einstein was asked to list the main awards he had received during his lifetime, he omitted the Nobel prize. |
|
|
|
The back-to-back Nobel prizes awarded to Guillaume and Einstein exemplifies an interesting fundamental dichotomy. Guillaume’s achievement has often been characterized as “routine” by the community of theoretical physicists, some of whom suggested that it wasn’t deserving of the Nobel – and yet it must be admitted that none of those theoreticians predicted the existence of anomalous behavior (the Invar effect) in metals. It was found only by Guillaume’s determined and practical-minded experimentation, and the practical benefits of his discovery have been immense. On the other hand, although Einstein and his theories of relativity were (and still are) revered by theoretical physicists, a good case could be made, at least for general relativity, that the direct practical benefits have been almost non-existent. It’s true that special relativity is fundamental to all of modern physics, to the extent that it’s hard to imagine modern physics without it, but in historical terms the special theory was, as Einstein himself said, “ripe for discovery in 1905”. (Needless to say, the splitting of the atom and the production of atomic bombs was primarily a product of the study of x-rays, radioactivity, and sub-atomic particles by people like Roentgen, Curie, and their successors, rather than a result of special relativity.) Whether quantum mechanics would have been hindered by the absence of the Einstein’s unique elucidation of the principles of special relativity is impossible to say. But it’s easy to see how his work on the photoelectric effect (which ironically was based on the experimental work of Philip Lenard, another Nobel prize winner and charter member of the Anti-Relativity Company Ltd.), together with his other work related to the light quanta, stimulated emission of radiation, and Einstein-Bose statistics, has been profoundly influential and facilitated the development of important technologies, including both television and the laser, with all the associated applications. Einstein’s theoretical work on relativity is comparable to the heliocentric theory of Copernicus, in the sense that the Copernican model did not lead directly to any practical benefits, and yet it stimulated and facilitated the entire scientific revolution. How then do we measure the “utility” of such fundamental theoretical ideas? Of course, even aside from utility, deep scientific truths possess a beauty that makes them highly valued by anyone with an appreciation for such things, but nothing needs to be said about that. |
|
|
|
Oddly enough, the two Guillaume cousins seem to have almost merged into a single individual in the scholarly literature. For example, in A. I. Miller’s book on the emergence of special relativity he cites “Charles Edouard Guillaume” as the author of the preface to the 1924 edition of Poincare’s “The New Mechanics”, in which the case is made for Poincare’s priority over Einstein, and for the Lorentz/Poincare ether-based approach. I’m fairly certain that this was actually written by Edouard Guillaume, the Swiss patent examiner who published so many anti-relativity papers, not by his cousin Charles Edouard Guillaume, the discoverer of Invar and winner of the Nobel prize. |
|
|
|
Gullstrand got his wish – up to a point. Einstein never did win a Nobel prize for relativity. However, half a century later, Nobel prizes were awarded in at least two cases for work related to general relativity, first for the detection of the cosmic microwave background radiation, related to the cosmological aspects of general relativity, and second (even more directly) for the analysis of pulsar evidence indicating the existence of gravitational radiation exactly in accord with Einstein’s field equations. Still, it’s safe to say that the incredibly precise instruments required to make those astronomical observations could not have been constructed without Invar and “the effect that defies understanding”. |
|
|